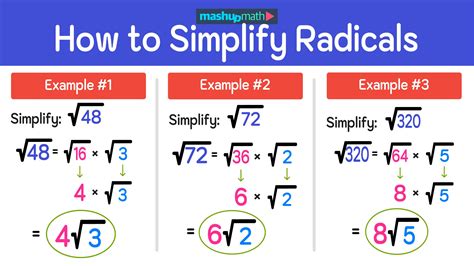
Simplifying radicals is an essential skill in mathematics, particularly in algebra and geometry. It involves expressing a radical expression in its simplest form, which can be beneficial for solving equations, graphing functions, and understanding mathematical concepts. In this article, we will explore the importance of simplifying radicals and provide a step-by-step guide on how to do it in 5 easy steps.
Simplifying radicals is crucial because it allows us to work with mathematical expressions more efficiently. When radicals are simplified, they become easier to manipulate and solve. This, in turn, can help us to better understand mathematical concepts and solve problems more effectively. Moreover, simplifying radicals is a fundamental skill that is required in various mathematical disciplines, including calculus, number theory, and algebra.
So, how do we simplify radicals? Let's dive into the 5 easy steps.
Step 1: Identify the Radical Expression

The first step in simplifying radicals is to identify the radical expression that needs to be simplified. A radical expression is an expression that contains a square root, cube root, or other root. It can be a simple expression, such as √(x), or a more complex expression, such as √(x^2 + 3x - 4). Once you have identified the radical expression, you can proceed to the next step.
What is a Radical Expression?
A radical expression is an expression that contains a root, such as a square root, cube root, or other root. It can be a simple expression, such as √(x), or a more complex expression, such as √(x^2 + 3x - 4). Radical expressions are used to represent quantities that cannot be expressed as simple integers or fractions.
Step 2: Factor the Radicand

The second step in simplifying radicals is to factor the radicand. The radicand is the expression inside the radical sign. Factoring the radicand involves breaking it down into its prime factors. This can be done using various factoring techniques, such as factoring out the greatest common factor (GCF), factoring by grouping, or using the quadratic formula.
Why Factor the Radicand?
Factoring the radicand is essential because it helps us to identify any perfect squares or perfect cubes that can be simplified. When we factor the radicand, we can identify any common factors that can be canceled out, which can simplify the radical expression.
Step 3: Identify Perfect Squares or Perfect Cubes

The third step in simplifying radicals is to identify any perfect squares or perfect cubes in the radicand. A perfect square is a number that can be expressed as the square of an integer, such as 16 = 4^2. A perfect cube is a number that can be expressed as the cube of an integer, such as 27 = 3^3. When we identify perfect squares or perfect cubes, we can simplify the radical expression by taking the square root or cube root of the perfect square or perfect cube.
What are Perfect Squares and Perfect Cubes?
Perfect squares and perfect cubes are numbers that can be expressed as the square or cube of an integer. Perfect squares are numbers that can be expressed as the square of an integer, such as 16 = 4^2. Perfect cubes are numbers that can be expressed as the cube of an integer, such as 27 = 3^3.
Step 4: Simplify the Radical Expression

The fourth step in simplifying radicals is to simplify the radical expression. This involves taking the square root or cube root of the perfect square or perfect cube and simplifying the resulting expression. When simplifying the radical expression, we can use various techniques, such as canceling out common factors, combining like terms, or using the quadratic formula.
How to Simplify a Radical Expression
To simplify a radical expression, we need to take the square root or cube root of the perfect square or perfect cube and simplify the resulting expression. This can be done by canceling out common factors, combining like terms, or using the quadratic formula.
Step 5: Check Your Answer

The final step in simplifying radicals is to check your answer. This involves verifying that the simplified radical expression is correct and that it satisfies the original equation. When checking your answer, you can use various techniques, such as plugging in values, graphing the function, or using algebraic manipulations.
Why Check Your Answer?
Checking your answer is essential because it helps you to verify that the simplified radical expression is correct and that it satisfies the original equation. This can help you to avoid errors and ensure that your solution is accurate.
By following these 5 easy steps, you can simplify radicals and make working with mathematical expressions more efficient. Remember to identify the radical expression, factor the radicand, identify perfect squares or perfect cubes, simplify the radical expression, and check your answer.
What is a radical expression?
+A radical expression is an expression that contains a root, such as a square root, cube root, or other root.
Why factor the radicand?
+Factoring the radicand helps us to identify any perfect squares or perfect cubes that can be simplified.
What are perfect squares and perfect cubes?
+Perfect squares and perfect cubes are numbers that can be expressed as the square or cube of an integer.
By simplifying radicals, you can make working with mathematical expressions more efficient and improve your understanding of mathematical concepts. Remember to practice regularly and use these 5 easy steps to simplify radicals with confidence.