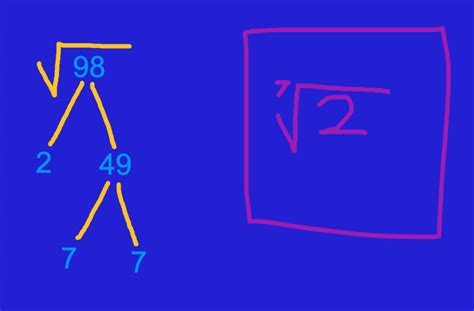
Simplifying the square root of 98 in radical form is a straightforward process that involves factoring the number under the radical sign into prime factors.
Simplifying Square Roots: Understanding the Basics

To simplify a square root, we need to find the largest perfect square that divides the number under the radical sign. A perfect square is a number that can be expressed as the square of an integer, such as 16 (4²) or 25 (5²). By factoring the number under the radical sign into prime factors, we can identify the perfect square factors and simplify the expression.
Factoring 98 into Prime Factors

To factor 98 into prime factors, we can start by dividing it by the smallest prime number, which is 2.
98 ÷ 2 = 49
Since 49 is not divisible by 2, we can try the next prime number, which is 3. However, 49 is not divisible by 3 either. Moving on to the next prime number, we find that 49 is divisible by 7.
49 ÷ 7 = 7
Therefore, the prime factorization of 98 is:
98 = 2 × 7 × 7
Simplifying the Square Root of 98

Now that we have factored 98 into prime factors, we can simplify the square root expression. We can take the square root of the perfect square factor, which is 7².
√98 = √(2 × 7 × 7) = √(7² × 2) = 7√2
Therefore, the simplified form of the square root of 98 in radical form is 7√2.
Example Problems
To further illustrate the concept of simplifying square roots in radical form, let's consider a few example problems.
Example 1: Simplify √50.
Solution:
√50 = √(2 × 5 × 5) = √(5² × 2) = 5√2
Example 2: Simplify √72.
Solution:
√72 = √(2 × 2 × 2 × 3 × 3) = √(2² × 3² × 2) = 2 × 3√2 = 6√2
Conclusion and Final Thoughts
Simplifying the square root of 98 in radical form involves factoring the number under the radical sign into prime factors and identifying the perfect square factors. By taking the square root of the perfect square factor, we can simplify the expression and write it in radical form. Remember to always factor the number under the radical sign into prime factors and identify the perfect square factors to simplify the expression.
What is the simplified form of the square root of 98 in radical form?
+The simplified form of the square root of 98 in radical form is 7√2.
How do you simplify a square root expression?
+To simplify a square root expression, you need to factor the number under the radical sign into prime factors and identify the perfect square factors. Then, take the square root of the perfect square factor to simplify the expression.
What is the prime factorization of 98?
+The prime factorization of 98 is 2 × 7 × 7.