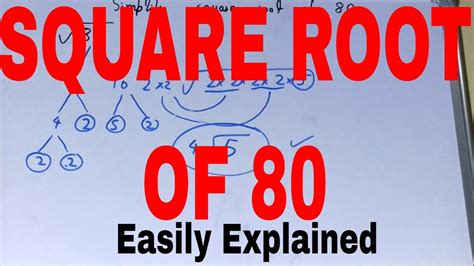
Simplifying radicals is an essential part of mathematics, especially when dealing with square roots. The square root of 80, denoted as √80, can be simplified using various methods. In this article, we will explore three ways to simplify the square root of 80 in radical form, ensuring that you have a solid grasp of the concept.
Simplifying radicals is a fundamental skill that can benefit students, educators, and professionals in various mathematical fields. Understanding how to break down complex radicals into simpler forms can enhance problem-solving skills and build a stronger foundation in mathematics. In this article, we will delve into three methods for simplifying the square root of 80, providing you with a comprehensive understanding of the process.
Method 1: Prime Factorization

One of the most effective methods for simplifying radicals is prime factorization. This method involves breaking down the number under the radical sign into its prime factors. To simplify √80 using prime factorization, we need to find the prime factors of 80.
80 = 2 × 2 × 2 × 2 × 5
Now, we can rewrite √80 as:
√80 = √(2 × 2 × 2 × 2 × 5)
Using the properties of radicals, we can simplify this expression:
√80 = √(2^4 × 5)
= 2^2 × √5
= 4√5
Therefore, the simplified radical form of √80 using prime factorization is 4√5.
Method 2: Finding Perfect Squares

Another method for simplifying radicals is finding perfect squares. This method involves identifying the largest perfect square that divides the number under the radical sign. To simplify √80 using this method, we need to find the largest perfect square that divides 80.
The largest perfect square that divides 80 is 16.
80 = 16 × 5
Now, we can rewrite √80 as:
√80 = √(16 × 5)
Using the properties of radicals, we can simplify this expression:
√80 = √16 × √5
= 4√5
Therefore, the simplified radical form of √80 using perfect squares is also 4√5.
Comparison of Methods
Both prime factorization and finding perfect squares are effective methods for simplifying radicals. However, prime factorization is often more efficient, especially when dealing with larger numbers. Finding perfect squares can be useful when the number under the radical sign is a product of a perfect square and another number.
Method 3: Using Radical Properties

A third method for simplifying radicals involves using radical properties. This method involves applying the properties of radicals to simplify the expression. To simplify √80 using radical properties, we can start by rewriting the expression as:
√80 = √(4 × 20)
Using the property of radicals that states √(a × b) = √a × √b, we can simplify this expression:
√80 = √4 × √20
= 2√20
Now, we can further simplify the expression by rewriting 20 as 4 × 5:
√80 = 2√(4 × 5)
= 2√4 × √5
= 4√5
Therefore, the simplified radical form of √80 using radical properties is also 4√5.
Conclusion
Simplifying radicals is an essential skill in mathematics, and there are various methods to achieve this. In this article, we explored three methods for simplifying the square root of 80 in radical form: prime factorization, finding perfect squares, and using radical properties. Each method has its advantages, and understanding these methods can help you simplify radicals with confidence.
Whether you're a student, educator, or professional, mastering the art of simplifying radicals can enhance your problem-solving skills and build a stronger foundation in mathematics. We encourage you to practice these methods and explore other techniques for simplifying radicals.
What is the simplified radical form of √80?
+The simplified radical form of √80 is 4√5.
What are the three methods for simplifying radicals discussed in this article?
+The three methods are prime factorization, finding perfect squares, and using radical properties.
Which method is often more efficient for simplifying radicals?
+Prime factorization is often more efficient, especially when dealing with larger numbers.