2 Easy Ways To Express √64 In Radical Form

Quick Links :

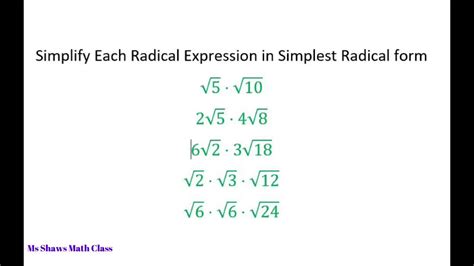
Simplifying radicals can seem like a daunting task, but with a few easy steps, you can master the process. In this article, we will explore two easy ways to express √64 in radical form. Whether you're a math enthusiast or just starting to learn about radicals, this article will guide you through the process with ease.

First, let's understand what radicals are. Radicals are a way of representing the nth root of a number. The symbol √ represents the square root, which is the 2nd root. In this case, we want to simplify √64. Before we dive into the methods, let's look at the importance of expressing radicals in their simplest form.

Expressing radicals in their simplest form is essential in mathematics, particularly in algebra and geometry. It helps in simplifying complex equations, making it easier to solve problems. Moreover, simplifying radicals enables us to identify common factors and simplify expressions more efficiently. Now, let's move on to the two easy ways to express √64 in radical form.

Method 1: Prime Factorization

One way to simplify √64 is by using prime factorization. Prime factorization involves breaking down a number into its prime factors. The prime factors of 64 are 2^6, since 2 x 2 x 2 x 2 x 2 x 2 = 64.

To simplify √64, we can rewrite it as √(2^6). Using the property of radicals that states √(a^2) = a, we can simplify further:

√(2^6) = √(2^4 x 2^2) = √(2^4) x √(2^2) = 2^2 x √(2^2) = 4 x √4 = 4 x 2 = 8

Therefore, √64 can be expressed as 8 in radical form.

Benefits of Prime Factorization

Prime factorization is a useful method for simplifying radicals because it allows us to identify the prime factors of a number. This method is particularly helpful when dealing with large numbers, as it enables us to break them down into smaller, more manageable factors.

Method 2: Finding Perfect Squares

Another way to simplify √64 is by finding perfect squares. A perfect square is a number that can be expressed as the square of an integer. In this case, we can rewrite 64 as 8^2, since 8 x 8 = 64.

Using the property of radicals that states √(a^2) = a, we can simplify further:

√(8^2) = 8

Therefore, √64 can also be expressed as 8 in radical form.

Benefits of Finding Perfect Squares

Finding perfect squares is a useful method for simplifying radicals because it allows us to identify numbers that can be expressed as the square of an integer. This method is particularly helpful when dealing with numbers that are already in a perfect square form, as it enables us to simplify them quickly and easily.

In conclusion, expressing √64 in radical form can be done using two easy methods: prime factorization and finding perfect squares. Both methods involve breaking down the number into its prime factors or identifying perfect squares, making it easier to simplify radicals. By mastering these methods, you can simplify radicals with ease and confidence.

We invite you to share your thoughts and questions in the comments section below. Have you used either of these methods to simplify radicals? Do you have any other methods to share? Share this article with your friends and classmates who may be struggling with simplifying radicals.