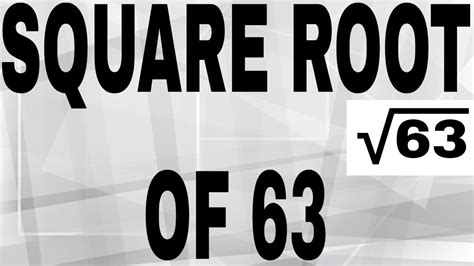
The concept of square roots is a fundamental part of mathematics, particularly in algebra and geometry. In this article, we will delve into the world of square roots, specifically focusing on the square root of 63 in radical form simplified.
Understanding Square Roots

A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16. Square roots can be expressed in various forms, including simplified radical form, which is a way of expressing square roots using the radical symbol (√).
What is Radical Form?
Radical form is a way of expressing square roots using the radical symbol (√). In this form, the square root of a number is expressed as the number inside the radical symbol, with the radical symbol indicating that it is a square root. For instance, the square root of 16 can be expressed as √16.
The Square Root of 63

Now, let's focus on the square root of 63. To simplify the square root of 63, we need to find the largest perfect square that divides 63. A perfect square is a number that can be expressed as the square of an integer, such as 1, 4, 9, 16, and so on.
Simplifying the Square Root of 63
The largest perfect square that divides 63 is 9. We can factor 63 as 9 × 7. Taking the square root of both sides, we get:
√63 = √(9 × 7)
Using the property of square roots that allows us to separate the square root of a product into the product of square roots, we can rewrite this as:
√63 = √9 × √7
Simplifying further, we get:
√63 = 3√7
Therefore, the square root of 63 in radical form simplified is 3√7.
Importance of Simplifying Square Roots

Simplifying square roots is an essential skill in mathematics, particularly in algebra and geometry. Simplifying square roots helps to:
- Reduce complex expressions to simpler forms
- Make calculations easier and more efficient
- Improve understanding of mathematical concepts
- Enhance problem-solving skills
Real-World Applications of Simplifying Square Roots
Simplifying square roots has numerous real-world applications, including:
- Physics and engineering, where square roots are used to calculate distances, velocities, and energies
- Computer science, where square roots are used in algorithms and data analysis
- Finance, where square roots are used in financial modeling and risk analysis
- Architecture, where square roots are used in designing buildings and structures
Conclusion and Final Thoughts

In conclusion, the square root of 63 in radical form simplified is 3√7. Simplifying square roots is an essential skill in mathematics, with numerous real-world applications. By understanding how to simplify square roots, we can improve our problem-solving skills, reduce complex expressions, and enhance our understanding of mathematical concepts.
We hope this article has provided you with a deeper understanding of square roots and their importance in mathematics. If you have any questions or comments, please feel free to share them below.
What is the square root of 63?
+The square root of 63 is √63 = 3√7.
Why is simplifying square roots important?
+Simplifying square roots is important because it helps to reduce complex expressions, make calculations easier, and improve understanding of mathematical concepts.
What are some real-world applications of simplifying square roots?
+Simplifying square roots has numerous real-world applications, including physics, engineering, computer science, finance, and architecture.