Finding Vertices In Factored Form Made Easy

Quick Links :

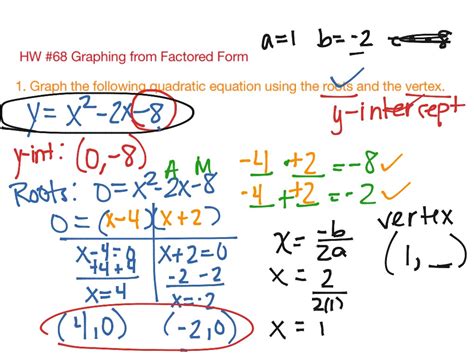
The vertex form of a quadratic function is a fundamental concept in algebra, and finding the vertices of a parabola can be a daunting task for many students. However, with the right approach and techniques, it can be made easy and even enjoyable. In this article, we will explore the concept of finding vertices in factored form, and provide practical examples and tips to help you master this skill.

Understanding the Vertex Form

The vertex form of a quadratic function is written as f(x) = a(x - h)^2 + k, where (h, k) represents the coordinates of the vertex. The vertex is the lowest or highest point on the parabola, depending on whether the parabola opens upwards or downwards. Understanding the vertex form is crucial to finding the vertices of a parabola.

Why is Finding Vertices Important?

Finding the vertices of a parabola has numerous applications in various fields, including physics, engineering, and computer science. In physics, the vertex of a parabola can represent the maximum or minimum height of a projectile, while in engineering, it can represent the maximum or minimum stress on a structure. In computer science, finding vertices is essential in graphics and game development.

Factored Form of a Quadratic Function

A quadratic function can be written in factored form as f(x) = a(x - p)(x - q), where p and q are the roots of the quadratic equation. The factored form is useful in finding the x-intercepts of the parabola, which are the points where the parabola intersects the x-axis.

How to Find Vertices in Factored Form

To find the vertices of a parabola in factored form, we need to follow these steps:

1. Identify the roots of the quadratic equation, p and q.
2. Find the midpoint of the roots, which is the x-coordinate of the vertex.
3. Substitute the x-coordinate of the vertex into the original equation to find the y-coordinate of the vertex.

Examples and Practice Problems

Let's consider a few examples to illustrate the process of finding vertices in factored form.

Example 1: Find the vertex of the parabola f(x) = (x - 2)(x + 3).

Solution: The roots of the quadratic equation are p = 2 and q = -3. The midpoint of the roots is x = (2 + (-3))/2 = -0.5. Substituting x = -0.5 into the original equation, we get f(-0.5) = (-0.5 - 2)(-0.5 + 3) = (-2.5)(2.5) = -6.25. Therefore, the vertex is (-0.5, -6.25).

Example 2: Find the vertex of the parabola f(x) = (x + 1)(x - 4).

Solution: The roots of the quadratic equation are p = -1 and q = 4. The midpoint of the roots is x = (-1 + 4)/2 = 1.5. Substituting x = 1.5 into the original equation, we get f(1.5) = (1.5 + 1)(1.5 - 4) = (2.5)(-2.5) = -6.25. Therefore, the vertex is (1.5, -6.25).

Tips and Tricks

Here are some tips and tricks to help you find vertices in factored form:

• Make sure to identify the roots of the quadratic equation correctly.
• Use the midpoint formula to find the x-coordinate of the vertex.
• Substitute the x-coordinate of the vertex into the original equation to find the y-coordinate of the vertex.
• Practice, practice, practice! The more you practice, the more comfortable you will become with finding vertices in factored form.

Common Mistakes to Avoid

Here are some common mistakes to avoid when finding vertices in factored form:

• Forgetting to find the roots of the quadratic equation.
• Using the wrong formula to find the midpoint of the roots.
• Failing to substitute the x-coordinate of the vertex into the original equation.
• Not simplifying the expression correctly.

Real-World Applications

Finding vertices in factored form has numerous real-world applications, including:

• Physics: Finding the maximum or minimum height of a projectile.
• Engineering: Finding the maximum or minimum stress on a structure.
• Computer Science: Finding the vertices of a polygon or a 3D model.

Conclusion

Finding vertices in factored form is a crucial skill in algebra, and with practice and patience, you can master it. Remember to identify the roots of the quadratic equation, find the midpoint of the roots, and substitute the x-coordinate of the vertex into the original equation. Avoid common mistakes and practice regularly to become proficient in finding vertices in factored form.

We hope this article has helped you understand the concept of finding vertices in factored form. If you have any questions or comments, please feel free to share them with us.