Slope Intercept Form For Parallel Lines Made Easy

Quick Links :

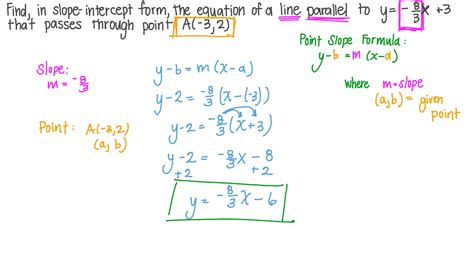
Understanding slope-intercept form is a fundamental concept in mathematics, particularly in algebra and geometry. It's a way to express the equation of a line in a specific format, making it easier to work with. In this article, we'll explore the concept of slope-intercept form, its significance, and how to use it to identify parallel lines.

What is Slope-Intercept Form?

Slope-intercept form is a way to write the equation of a line in the format y = mx + b, where:

• m is the slope of the line
• b is the y-intercept (the point where the line intersects the y-axis)
• x is the independent variable
• y is the dependent variable

The slope-intercept form is useful because it provides information about the line's steepness (slope) and its position on the coordinate plane (y-intercept).

Why is Slope-Intercept Form Important?

The slope-intercept form is essential in mathematics and real-world applications because it:

• Helps to identify the line's slope and y-intercept
• Enables you to graph the line easily
• Facilitates the calculation of the line's equation
• Allows for the comparison of lines to determine if they are parallel, perpendicular, or neither

Identifying Parallel Lines using Slope-Intercept Form

Parallel lines are lines that lie in the same plane and never intersect, no matter how far they are extended. To identify parallel lines using slope-intercept form, you need to compare their slopes.

• If two lines have the same slope (m), they are parallel.
• If two lines have different slopes, they are not parallel.

For example, consider the following equations:

Line 1: y = 2x + 3 Line 2: y = 2x + 5

Both lines have the same slope (m = 2), so they are parallel.

Steps to Identify Parallel Lines using Slope-Intercept Form

Here are the steps to follow:

1. Write the equations of the lines in slope-intercept form (y = mx + b).
2. Compare the slopes (m) of the lines.
3. If the slopes are the same, the lines are parallel.
4. If the slopes are different, the lines are not parallel.

Real-World Applications of Slope-Intercept Form

Slope-intercept form has numerous real-world applications in various fields, including:

• Physics: to describe the motion of objects
• Engineering: to design and analyze systems
• Computer Science: to create algorithms and models
• Economics: to model economic systems and trends

For instance, in physics, the slope-intercept form can be used to describe the motion of an object under constant acceleration. The slope represents the acceleration, and the y-intercept represents the initial velocity.

Practical Examples of Slope-Intercept Form

Here are some practical examples of slope-intercept form:

• A company's profit can be modeled using the equation P = 2x + 1000, where P is the profit, x is the number of units sold, and 2 is the slope (representing the rate of change of profit).
• A car's distance traveled can be modeled using the equation d = 50t + 20, where d is the distance, t is the time, and 50 is the slope (representing the speed).

Conclusion

In conclusion, slope-intercept form is a powerful tool for working with linear equations and identifying parallel lines. By understanding the concept of slope-intercept form and its applications, you can solve problems in various fields and make informed decisions.

Call to Action

We hope this article has helped you understand slope-intercept form and its significance in mathematics and real-world applications. If you have any questions or need further clarification, please leave a comment below. Share this article with your friends and colleagues who may find it helpful.