Geometric Series Closed Form Formula Explained Simply

Quick Links :

Mathematics has always been a fundamental part of human understanding and innovation. From the intricate patterns found in nature to the complex algorithms that govern modern technology, mathematical concepts have played a pivotal role in shaping our world. One such concept that has far-reaching implications in various fields is the geometric series. In this article, we will delve into the world of geometric series, exploring its definition, the closed-form formula, and practical applications, making the complex simple and accessible.

A geometric series is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio. This simple yet powerful concept has been used to model population growth, compound interest, and even the spread of diseases. However, working with geometric series can become cumbersome when dealing with large numbers of terms. This is where the geometric series closed-form formula comes into play, providing a concise and efficient way to calculate the sum of a geometric series.

Understanding Geometric Series

To grasp the geometric series formula, let's first understand what a geometric series looks like. Consider a series where the first term, denoted as (a), is 2, and the common ratio, denoted as (r), is 3. The series would start as 2, 6, 18, 54, and so on. Notice how each term is obtained by multiplying the previous term by the common ratio, in this case, 3.

Elements of a Geometric Series

• First Term (a): The starting value of the series.
• Common Ratio (r): The number by which each term is multiplied to get the next term.
• Number of Terms (n): The total count of terms in the series.

The Geometric Series Closed-Form Formula

The closed-form formula for the sum of a geometric series is given by:

[ S_n = a \times \frac{1 - r^n}{1 - r} ]

where (S_n) is the sum of the first (n) terms, (a) is the first term, (r) is the common ratio, and (n) is the number of terms.

This formula is a powerful tool that allows us to calculate the sum of a geometric series without having to add each term individually, which can be especially useful when dealing with a large number of terms.

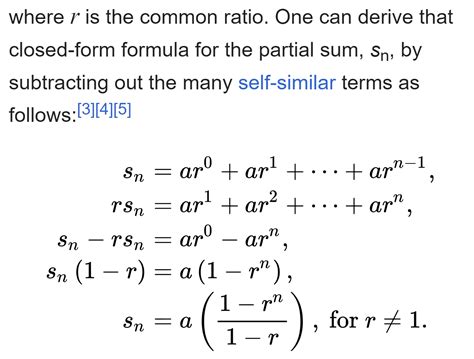
Derivation of the Formula

To derive the formula, let's consider a geometric series with (n) terms:

[ S_n = a + ar + ar^2 + \cdots + ar^{n-1} ]

Multiplying both sides by (r), we get:

[ rS_n = ar + ar^2 + \cdots + ar^{n-1} + ar^n ]

Subtracting the first equation from the second gives:

[ rS_n - S_n = ar^n - a ]

Factoring (S_n) and rearranging terms, we obtain the formula:

[ S_n = a \times \frac{1 - r^n}{1 - r} ]

Practical Applications of Geometric Series

Geometric series have numerous applications in various fields, including:

• Compound Interest: Banks use geometric series to calculate the future value of an investment based on the principal amount, interest rate, and time.
• Population Growth: Geometric series can model the growth of a population over time, assuming a constant birth rate and no deaths or emigration.
• Disease Spread: The spread of a disease can be modeled using a geometric series, taking into account the number of infected individuals and the rate of transmission.

Calculating Compound Interest

Suppose you deposit $1,000 into a savings account that earns a 5% annual interest rate. Using the geometric series formula, you can calculate the future value of your investment after 5 years:

[ S_5 = 1000 \times \frac{1 - (1.05)^5}{1 - 1.05} ]

[ S_5 = 1000 \times \frac{1 - 1.2762815625}{-0.05} ]

[ S_5 = 1000 \times \frac{-0.2762815625}{-0.05} ]

[ S_5 = 1000 \times 5.52563125 ]

[ S_5 = 5525.63125 ]

So, after 5 years, your investment would be worth approximately $5,525.63.

Conclusion - Embracing the Power of Geometric Series

In conclusion, geometric series and their closed-form formula offer a powerful tool for solving problems in various fields. By understanding the concept and application of geometric series, you can simplify complex calculations and gain insights into the world around you. Whether you're a student looking to grasp mathematical concepts or a professional seeking to apply them in real-world scenarios, the geometric series formula is an invaluable resource to have in your toolkit.

We hope this article has provided you with a comprehensive understanding of geometric series and their applications. Do you have any questions or topics you'd like to discuss further? Please leave a comment below and share your thoughts with us!