Simplifying radical forms is an essential skill in mathematics, particularly in algebra and geometry. Radical forms, also known as radicals, are mathematical expressions that contain a square root or other root symbol. Simplifying these expressions can make them easier to work with and understand. In this article, we will explore five ways to simplify radical forms, along with practical examples and explanations.
What are Radical Forms?
Before we dive into simplifying radical forms, let's quickly review what they are. A radical form is a mathematical expression that contains a root symbol, such as a square root (√), cube root (∛), or nth root (∜). For example, √x, ∛y, and ∜z are all radical forms.
Method 1: Simplifying Square Roots
One of the most common types of radical forms is the square root. To simplify a square root, we need to find the largest perfect square that divides the number inside the square root.

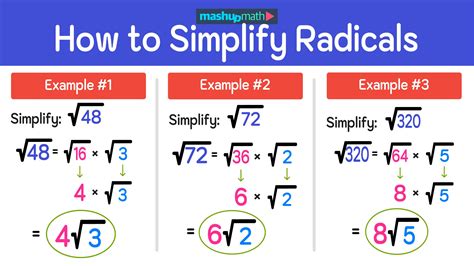
For example, let's simplify √48. We can start by finding the largest perfect square that divides 48. In this case, it's 16. So, we can rewrite √48 as √(16 × 3) = 4√3.
Method 2: Simplifying Cube Roots
Cube roots are similar to square roots, but they involve finding the cube of a number instead of the square. To simplify a cube root, we need to find the largest perfect cube that divides the number inside the cube root.

For example, let's simplify ∛54. We can start by finding the largest perfect cube that divides 54. In this case, it's 27. So, we can rewrite ∛54 as ∛(27 × 2) = 3∛2.
Method 3: Simplifying nth Roots
nth roots are a generalization of square roots and cube roots. To simplify an nth root, we need to find the largest perfect nth power that divides the number inside the nth root.

For example, let's simplify ∜80. We can start by finding the largest perfect fourth power that divides 80. In this case, it's 16. So, we can rewrite ∜80 as ∜(16 × 5) = 2∜5.
Method 4: Simplifying Radical Expressions with Variables
Radical forms can also involve variables. To simplify these expressions, we need to find the largest perfect power that divides the coefficient of the variable.

For example, let's simplify √(16x^2). We can start by finding the largest perfect square that divides 16. In this case, it's 16. So, we can rewrite √(16x^2) as 4x.
Method 5: Simplifying Radical Expressions with Fractions
Radical forms can also involve fractions. To simplify these expressions, we need to rationalize the denominator by multiplying both the numerator and denominator by the conjugate of the denominator.

For example, let's simplify 1 / √2. We can start by multiplying both the numerator and denominator by the conjugate of the denominator, which is √2. So, we can rewrite 1 / √2 as (√2) / 2.
Conclusion
Simplifying radical forms is an essential skill in mathematics. By using these five methods, you can simplify square roots, cube roots, nth roots, radical expressions with variables, and radical expressions with fractions. Remember to always find the largest perfect power that divides the number inside the radical form, and to rationalize the denominator when working with fractions.
Take Action!
We hope this article has helped you to understand the different methods for simplifying radical forms. Try practicing these methods with different examples, and see how they can help you to simplify complex mathematical expressions. If you have any questions or comments, please feel free to share them below.
Frequently Asked Questions
What is the difference between a square root and a cube root?
+A square root is a mathematical expression that finds the number that, when multiplied by itself, gives a specified value. A cube root is a mathematical expression that finds the number that, when multiplied by itself twice, gives a specified value.
How do I simplify a radical expression with a variable?
+To simplify a radical expression with a variable, find the largest perfect power that divides the coefficient of the variable. Then, rewrite the expression using the simplified coefficient.
Can I simplify a radical expression with a fraction by multiplying both the numerator and denominator by the conjugate of the denominator?
+Yes, you can simplify a radical expression with a fraction by multiplying both the numerator and denominator by the conjugate of the denominator. This will rationalize the denominator and simplify the expression.