Simplify 180 To Simplest Radical Form In 5 Steps

Quick Links :

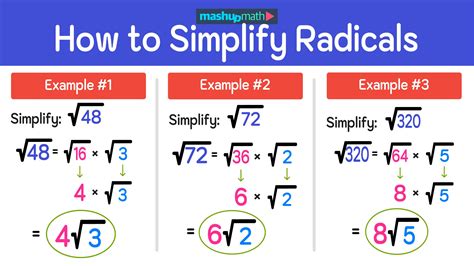
Simplifying Radicals: A 5-Step Guide to Simplify 180 to Simplest Radical Form

Radicals, also known as roots, are an essential concept in mathematics, particularly in algebra and geometry. Simplifying radicals is a crucial skill to master, as it helps in solving equations and problems involving square roots, cube roots, and other types of roots. In this article, we will focus on simplifying the radical expression 180 to its simplest form using a 5-step approach.

Step 1: Factor the Number Inside the Radical

To simplify the radical expression 180, we need to start by factoring the number inside the radical sign (√). Factoring 180, we get:

180 = 2 × 2 × 3 × 3 × 5

Step 2: Identify Perfect Squares

Next, we need to identify perfect squares within the factors. A perfect square is a number that can be expressed as the square of an integer. In this case, we have:

2 × 2 = 4 (perfect square) 3 × 3 = 9 (perfect square)

Step 3: Take Out the Perfect Squares

Now, we can take out the perfect squares from under the radical sign:

√180 = √(4 × 9 × 5) = √(4) × √(9) × √5 = 2 × 3 × √5

Step 4: Simplify the Radical Expression

By taking out the perfect squares, we have simplified the radical expression:

√180 = 2 × 3 × √5 = 6√5

Step 5: Check for Further Simplification

Finally, we need to check if the radical expression can be further simplified. In this case, the expression 6√5 is already in its simplest form, as there are no more perfect squares or other simplifications possible.

Benefits of Simplifying Radicals

Simplifying radicals has several benefits in mathematics and real-world applications. Some of the advantages include:

• Easier calculations: Simplifying radicals makes calculations easier and faster, as it reduces the complexity of the expression.
• Improved accuracy: Simplifying radicals helps to reduce errors and improves accuracy in mathematical calculations.
• Enhanced problem-solving: Simplifying radicals is essential in solving equations and problems involving radicals, which is critical in various fields, such as physics, engineering, and computer science.

Common Mistakes to Avoid When Simplifying Radicals

When simplifying radicals, it's essential to avoid common mistakes that can lead to incorrect results. Some of the mistakes to watch out for include:

• Forgetting to check for perfect squares: Failing to identify perfect squares within the factors can lead to incomplete simplification.
• Incorrectly taking out perfect squares: Taking out perfect squares incorrectly can result in an incorrect simplified expression.
• Not checking for further simplification: Failing to check for further simplification can lead to an expression that is not in its simplest form.

Conclusion

In conclusion, simplifying radicals is an essential skill in mathematics, and following the 5-step approach outlined in this article can help to simplify radical expressions like 180 to their simplest form. By factoring the number inside the radical, identifying perfect squares, taking out perfect squares, simplifying the radical expression, and checking for further simplification, you can ensure that your radical expressions are simplified correctly.

We hope this article has been informative and helpful in your journey to master simplifying radicals. If you have any questions or comments, please feel free to share them below.

Take Action:

• Share this article with your friends and classmates who may benefit from learning about simplifying radicals.
• Practice simplifying radicals using the 5-step approach outlined in this article.
• Explore other topics in mathematics, such as algebra and geometry, and learn how simplifying radicals can help in solving problems and equations.

FAQ Section: