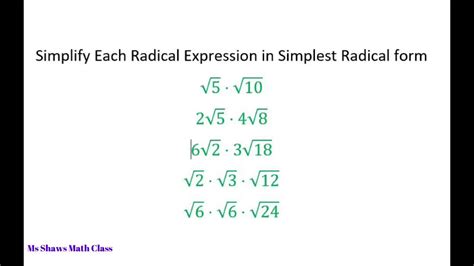
Solving For X In Simplest Radical Form Made Easy

Quick Links :

Solving for x in simplest radical form is a fundamental concept in algebra, and it's essential to understand the process to succeed in mathematics. In this article, we'll break down the steps to solve for x in simplest radical form, making it easy and accessible for everyone.

Radicals are an integral part of algebra, and they can be intimidating at first. However, with a clear understanding of the concept and the steps involved, you'll be solving equations like a pro in no time. So, let's dive into the world of radicals and explore how to solve for x in simplest radical form.

What are Radicals?

Radicals are mathematical expressions that contain a radical sign (√). The radical sign indicates that we're dealing with the root of a number. For example, √16 represents the square root of 16, which is 4. Radicals can be simplified by finding the factors of the number under the radical sign.

Types of Radicals

There are two main types of radicals:

• Square roots (√)
• Cube roots (∛)
• Higher-order roots (e.g., fourth roots, fifth roots, etc.)

Solving for x in Simplest Radical Form

To solve for x in simplest radical form, follow these steps:

1. Isolate the Radical: Isolate the radical expression on one side of the equation.
2. Find the Factor: Find the factor of the number under the radical sign.
3. Simplify the Radical: Simplify the radical expression by finding the square root, cube root, or higher-order root.
4. Check for Rationalization: Check if the expression needs to be rationalized (i.e., removing the radical sign).

Example 1: Square Root

Solve for x: √x = 4

• Isolate the Radical: √x = 4
• Find the Factor: x = 16
• Simplify the Radical: x = √16
• Check for Rationalization: x = 4 (no rationalization needed)

Step-by-Step Guide to Solving Radicals

Here's a step-by-step guide to solving radicals:

1. Identify the Type of Radical: Determine if it's a square root, cube root, or higher-order root.
2. Find the Factor: Find the factors of the number under the radical sign.
3. Simplify the Radical: Simplify the radical expression using the factors found.
4. Rationalize the Expression: Rationalize the expression, if necessary.
5. Check for Errors: Check the solution for errors and ensure it's in simplest radical form.

Example 2: Cube Root

Solve for x: ∛x = 2

• Identify the Type of Radical: Cube root
• Find the Factor: x = 8
• Simplify the Radical: x = ∛8
• Rationalize the Expression: x = 2 (no rationalization needed)
• Check for Errors: x = 2 (correct solution)

Common Mistakes to Avoid

When solving for x in simplest radical form, avoid these common mistakes:

• Forgetting to rationalize the expression
• Incorrectly identifying the type of radical
• Failing to check for errors

Conclusion: Solving for x in Simplest Radical Form

Solving for x in simplest radical form requires attention to detail and a clear understanding of the steps involved. By following the steps outlined in this article and practicing regularly, you'll become proficient in solving radicals and tackling more complex mathematical problems.

Unlock Your Math Potential

Don't let radicals intimidate you! With the right guidance and practice, you can unlock your math potential and become a master of solving for x in simplest radical form.

Now, it's your turn! Share your experiences, ask questions, or provide feedback in the comments section below.