Simplify Fractions In 2 Easy Steps

Quick Links :

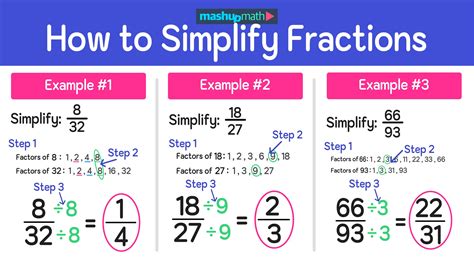
Simplifying fractions is a fundamental math skill that can be a challenge for many students. However, with the right approach, it can be a straightforward process. In this article, we will explore the importance of simplifying fractions, the benefits of doing so, and provide a step-by-step guide on how to simplify fractions in 2 easy steps.

Why Simplify Fractions?

Simplifying fractions is essential in mathematics because it helps to:

• Reduce complexity: Simplifying fractions makes them easier to work with, especially when adding, subtracting, multiplying, or dividing them.
• Improve accuracy: Simplified fractions reduce the likelihood of errors when performing mathematical operations.
• Enhance understanding: Simplifying fractions helps to reveal the underlying structure of the fraction, making it easier to understand and work with.

Benefits of Simplifying Fractions

Simplifying fractions has several benefits, including:

• Improved problem-solving skills: Simplified fractions make it easier to solve math problems, especially in algebra and geometry.
• Enhanced math fluency: Simplifying fractions helps to build math fluency, making it easier to perform mathematical operations quickly and accurately.
• Better understanding of math concepts: Simplified fractions help to reveal the underlying math concepts, making it easier to understand and apply them.

Step 1: Find the Greatest Common Divisor (GCD)

The first step in simplifying fractions is to find the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both the numerator and denominator without leaving a remainder.

To find the GCD, you can use the following methods:

• List the factors of the numerator and denominator and find the greatest common factor.
• Use the Euclidean algorithm to find the GCD.
• Use online tools or calculators to find the GCD.

Example: Find the GCD of 12 and 18

Factors of 12: 1, 2, 3, 4, 6, 12 Factors of 18: 1, 2, 3, 6, 9, 18

GCD: 6

Step 2: Divide the Numerator and Denominator by the GCD

Once you have found the GCD, you can simplify the fraction by dividing the numerator and denominator by the GCD.

Example: Simplify the Fraction 12/18

GCD: 6

Divide the numerator and denominator by the GCD:

12 ÷ 6 = 2 18 ÷ 6 = 3

Simplified fraction: 2/3

Practical Examples

Here are some practical examples of simplifying fractions:

• Simplify the fraction 4/8:

GCD: 4

Divide the numerator and denominator by the GCD:

4 ÷ 4 = 1 8 ÷ 4 = 2

Simplified fraction: 1/2

• Simplify the fraction 9/12:

GCD: 3

Divide the numerator and denominator by the GCD:

9 ÷ 3 = 3 12 ÷ 3 = 4

Simplified fraction: 3/4

Conclusion

Simplifying fractions is a straightforward process that can be achieved in 2 easy steps. By finding the greatest common divisor (GCD) and dividing the numerator and denominator by the GCD, you can simplify fractions and make them easier to work with. Remember to practice simplifying fractions regularly to build your math fluency and enhance your problem-solving skills.

We hope this article has been helpful in explaining the importance of simplifying fractions and providing a step-by-step guide on how to do so. If you have any questions or comments, please feel free to share them below.