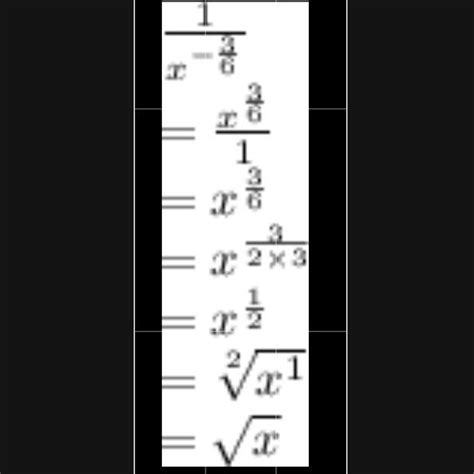
Rewriting expressions in simplest radical form is an essential skill in algebra and mathematics. It involves simplifying expressions that contain radicals, such as square roots, cube roots, and fourth roots, among others. The goal is to express these radicals in their simplest form, which makes them easier to work with and understand. Here are three steps to rewrite expressions in simplest radical form:

Understanding Radicals
Before we dive into the steps to rewrite expressions in simplest radical form, it's essential to understand what radicals are and how they work. A radical is a symbol that represents the root of a number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16. Radicals can be square roots, cube roots, fourth roots, and so on.
Types of Radicals
There are several types of radicals, including:
- Square roots: represented by the symbol √
- Cube roots: represented by the symbol ³√
- Fourth roots: represented by the symbol ⁴√
- nth roots: represented by the symbol ⁿ√
Step 1: Factor Out Perfect Squares
The first step to rewrite an expression in simplest radical form is to factor out perfect squares. A perfect square is a number that can be expressed as the square of an integer. For example, 16 is a perfect square because it can be expressed as 4².

To factor out perfect squares, look for numbers that can be expressed as the square of an integer. For example, if you have the expression √16, you can factor out the perfect square 4², which equals 16.
Example
√16 = √(4²) = 4
Step 2: Simplify the Radical
The second step to rewrite an expression in simplest radical form is to simplify the radical. To simplify a radical, look for any common factors between the radicand (the number inside the radical) and the index (the number outside the radical).

For example, if you have the expression ³√8, you can simplify the radical by looking for common factors between the radicand 8 and the index 3. In this case, the greatest common factor is 2, so you can simplify the radical as follows:
³√8 = ³√(2³) = 2
Example
³√8 = ³√(2³) = 2
Step 3: Check for Any Remaining Radicals
The third and final step to rewrite an expression in simplest radical form is to check for any remaining radicals. If there are any remaining radicals, you may need to repeat the process of factoring out perfect squares and simplifying the radical.

For example, if you have the expression √(16 + 9), you can factor out the perfect square 16 and simplify the radical as follows:
√(16 + 9) = √(4² + 3²) = √(4 + 3)² = 4 + 3 = 5
However, this expression can be further simplified by factoring out the perfect square 25:
√(16 + 9) = √(4² + 3²) = √(4 + 3)² = √25 = 5
Example
√(16 + 9) = √(4² + 3²) = √(4 + 3)² = √25 = 5
Now that you've completed the three steps to rewrite an expression in simplest radical form, you should be able to simplify even the most complex radical expressions.
What is a radical in mathematics?
+A radical is a symbol that represents the root of a number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16.
What is the simplest radical form of √16?
+The simplest radical form of √16 is 4, because 4 multiplied by 4 equals 16.
How do I simplify a radical expression?
+To simplify a radical expression, factor out perfect squares, simplify the radical, and check for any remaining radicals.
We hope this article has helped you understand the three steps to rewrite expressions in simplest radical form. If you have any questions or need further clarification, please don't hesitate to ask.