Simplify Radical 128 In 5 Easy Steps

Quick Links :

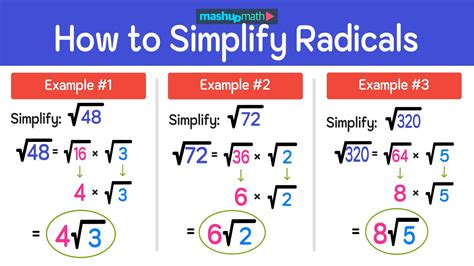
Radical numbers can be intimidating, but simplifying them can be a breeze with the right approach. In this article, we'll break down the process of simplifying radical 128 into 5 easy steps.

What is Radical 128?

Radical 128, also known as the square root of 128, is a mathematical expression that represents the value that, when multiplied by itself, gives 128. It's denoted by the symbol √128.

Why Simplify Radical 128?

Simplifying radical 128 is essential in various mathematical operations, such as algebra, geometry, and calculus. By simplifying radical 128, you can make calculations easier, reduce errors, and gain a deeper understanding of mathematical concepts.

Step 1: Factor Out Perfect Squares

The first step in simplifying radical 128 is to factor out perfect squares. A perfect square is a number that can be expressed as the square of an integer. In this case, 128 can be factored as 64 × 2, where 64 is a perfect square (8^2).

Step 2: Simplify the Perfect Square

Now that we've factored out the perfect square, we can simplify it. The square root of 64 is 8, since 8^2 = 64.

Step 3: Simplify the Remaining Radical

We're left with √2, which cannot be simplified further. However, we can express it as a product of two square roots: √2 = √(2 × 1) = √2 × √1.

Step 4: Combine the Simplified Radicals

Now, we combine the simplified radicals: 8√2. This is the simplified form of radical 128.

Step 5: Verify the Simplification

To verify our simplification, we can multiply 8√2 by itself and check if it equals 128: (8√2)^2 = 64 × 2 = 128. This confirms that our simplification is correct.

Practical Applications of Simplifying Radical 128

Simplifying radical 128 has various practical applications in mathematics, science, and engineering. For instance, it's used in calculating distances, velocities, and accelerations in physics and engineering problems.

Benefits of Simplifying Radical 128

Simplifying radical 128 offers several benefits, including:

• Easier calculations: Simplifying radical 128 reduces the complexity of mathematical operations, making calculations faster and more accurate.
• Reduced errors: By simplifying radical 128, you minimize the risk of errors that can occur when working with complex radical expressions.
• Deeper understanding: Simplifying radical 128 helps you understand mathematical concepts more deeply, making it easier to apply them to real-world problems.

Common Mistakes to Avoid

When simplifying radical 128, it's essential to avoid common mistakes, such as:

• Incorrect factoring: Make sure to factor out perfect squares correctly to avoid errors.
• Incomplete simplification: Ensure that you simplify the radical completely, without leaving any square roots unsimplified.

Conclusion: Take the First Step Towards Mathematical Mastery

Simplifying radical 128 is a fundamental skill that can help you master mathematical concepts and solve complex problems with ease. By following the 5 easy steps outlined in this article, you can simplify radical 128 and take the first step towards mathematical mastery.

What's your experience with simplifying radical numbers? Share your thoughts, ask questions, or provide examples in the comments below!