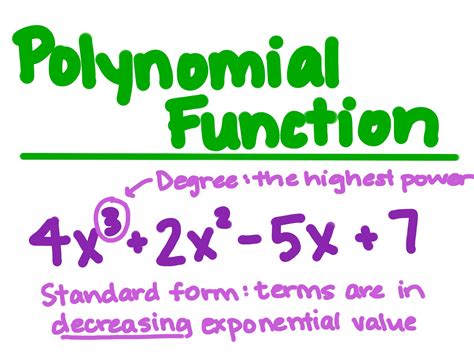
Polynomials In Standard Form Explained

Quick Links :

Polynomials are a fundamental concept in algebra, and understanding their standard form is crucial for working with them effectively. In this article, we will delve into the world of polynomials, exploring what they are, their importance, and how to express them in standard form.

Polynomials are mathematical expressions consisting of variables and coefficients combined using only addition, subtraction, and multiplication. They are used to model a wide range of real-world phenomena, from the growth of populations to the motion of objects. The versatility of polynomials makes them an essential tool in various fields, including physics, engineering, economics, and computer science.

What is Standard Form?

The standard form of a polynomial is a way of expressing it in a specific format, which makes it easier to work with and analyze. In standard form, the polynomial is written in descending order of exponents, with the term having the highest exponent first. This format is also known as the "general form" or "expanded form" of a polynomial.

Example of a Polynomial in Standard Form

Consider the polynomial 3x^2 + 2x - 4. In this example, the term with the highest exponent (x^2) comes first, followed by the term with the next highest exponent (x), and finally the constant term (-4). This is the standard form of the polynomial.

Benefits of Standard Form

Expressing polynomials in standard form has several benefits, including:

• Easy comparison: Standard form makes it easy to compare two or more polynomials, as the terms are arranged in a consistent order.
• Simplification: Standard form allows for easy simplification of polynomials, as like terms can be combined.
• Analysis: Standard form facilitates the analysis of polynomials, as the coefficients and exponents are clearly visible.

Steps to Write a Polynomial in Standard Form

To write a polynomial in standard form, follow these steps:

1. Identify the terms of the polynomial and their corresponding exponents.
2. Arrange the terms in descending order of exponents.
3. Combine like terms, if any.
4. Write the polynomial in the standard form format.

Types of Polynomials

Polynomials can be classified into several types, including:

• Monomials: Polynomials with only one term.
• Binomials: Polynomials with two terms.
• Trinomials: Polynomials with three terms.
• Quadratics: Polynomials with a degree of two.

Real-World Applications of Polynomials

Polynomials have numerous real-world applications, including:

• Physics: Polynomials are used to model the motion of objects, including the trajectory of projectiles and the vibration of springs.
• Engineering: Polynomials are used to design electronic circuits and model the behavior of complex systems.
• Economics: Polynomials are used to model economic systems and make predictions about future trends.

Conclusion and Final Thoughts

In conclusion, polynomials are a fundamental concept in algebra, and expressing them in standard form is crucial for working with them effectively. By understanding the benefits and steps to write a polynomial in standard form, you can improve your skills in algebra and apply them to real-world problems. We hope this article has provided you with a comprehensive understanding of polynomials in standard form.

We invite you to share your thoughts and questions about polynomials in standard form in the comments section below. If you found this article helpful, please share it with your friends and colleagues.