Convert Polynomial To Factored Form Easily

Quick Links :

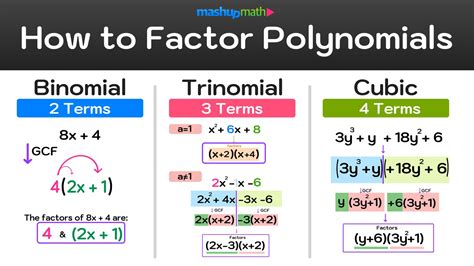
Converting polynomials to factored form is a fundamental concept in algebra, and it can seem daunting at first. However, with the right techniques and strategies, you can easily simplify polynomials and express them in factored form. In this article, we will explore the importance of factoring polynomials, the benefits of factoring, and provide step-by-step guides on how to convert polynomials to factored form.

Why is Factoring Polynomials Important?

Factoring polynomials is a crucial skill in algebra, as it allows you to simplify complex expressions, solve equations, and graph functions. Factoring polynomials can help you:

• Simplify complex expressions by breaking them down into simpler components
• Solve equations by isolating variables and finding roots
• Graph functions by identifying key features such as x-intercepts and turning points
• Identify patterns and relationships between variables

Benefits of Factoring Polynomials

Factoring polynomials has numerous benefits, including:

• Improved problem-solving skills: Factoring polynomials can help you develop critical thinking and problem-solving skills.
• Enhanced understanding of algebraic concepts: Factoring polynomials can help you understand key algebraic concepts, such as quadratic equations and functions.
• Increased confidence: Factoring polynomials can help you build confidence in your math abilities and tackle more complex problems.

Step-by-Step Guide to Factoring Polynomials

Factoring polynomials involves several steps, including:

Step 1: Identify the Type of Polynomial

Identify the type of polynomial you are working with, such as quadratic, cubic, or quartic.

Step 2: Look for Common Factors

Look for common factors among the terms, such as greatest common factors (GCFs) or common binomial factors.

Step 3: Factor by Grouping

Group terms that have common factors and factor them out.

Step 4: Factor Quadratic Expressions

Use techniques such as the quadratic formula or completing the square to factor quadratic expressions.

Step 5: Factor Cubic and Quartic Expressions

Use techniques such as the rational root theorem or synthetic division to factor cubic and quartic expressions.

Factoring Quadratic Expressions

Factoring quadratic expressions involves finding two binomials whose product is the quadratic expression.

• Example: Factor x^2 + 5x + 6
• Solution: (x + 3)(x + 2)

Factoring Cubic and Quartic Expressions

Factoring cubic and quartic expressions involves finding a combination of linear and quadratic factors.

• Example: Factor x^3 + 2x^2 - 7x - 12
• Solution: (x + 3)(x^2 - x - 4)

Tips and Tricks for Factoring Polynomials

Here are some tips and tricks to help you factor polynomials:

• Look for patterns and relationships between terms
• Use the quadratic formula or completing the square to factor quadratic expressions
• Use the rational root theorem or synthetic division to factor cubic and quartic expressions
• Practice, practice, practice!

Common Mistakes to Avoid

Here are some common mistakes to avoid when factoring polynomials:

• Forgetting to check for common factors
• Using the wrong technique for the type of polynomial
• Making errors in calculations

Conclusion

Factoring polynomials is a fundamental concept in algebra, and with the right techniques and strategies, you can easily simplify polynomials and express them in factored form. Remember to identify the type of polynomial, look for common factors, and use techniques such as the quadratic formula or completing the square to factor quadratic expressions. Practice makes perfect, so keep practicing and you will become a pro at factoring polynomials in no time!