7 Point Slope Form Problems To Try Now

Quick Links :

Hide

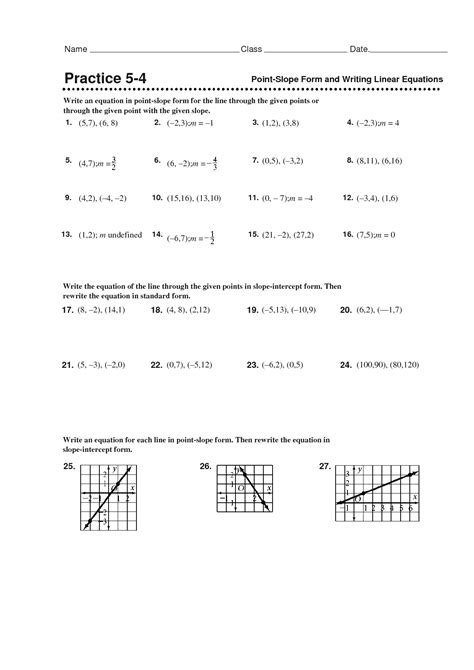
Are you ready to put your understanding of point-slope form to the test? Here are 7 problems to help you practice and solidify your skills.

What is Point-Slope Form? Before we dive into the problems, let's quickly review what point-slope form is. Point-slope form is a way of writing a linear equation in the form y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope.

Problem 1: Writing in Point-Slope Form Write the equation of the line that passes through the point (2, 3) and has a slope of 4.

Solution

Using the point-slope form formula, we get y - 3 = 4(x - 2). Simplifying this equation, we get y = 4x - 5.

Problem 2: Finding the Slope Find the slope of the line that passes through the points (1, 2) and (3, 4).

Solution

To find the slope, we can use the formula m = (y2 - y1) / (x2 - x1). Plugging in the values, we get m = (4 - 2) / (3 - 1) = 1.

Problem 3: Writing in Point-Slope Form Write the equation of the line that passes through the point (-1, 2) and has a slope of -3.

Solution

Using the point-slope form formula, we get y - 2 = -3(x - (-1)). Simplifying this equation, we get y = -3x - 1.

Problem 4: Finding the Equation Find the equation of the line that passes through the points (2, 1) and (4, 3).

Solution

To find the equation, we can use the point-slope form formula. First, we need to find the slope using the formula m = (y2 - y1) / (x2 - x1). Plugging in the values, we get m = (3 - 1) / (4 - 2) = 1. Then, we can use the point-slope form formula to get y - 1 = 1(x - 2). Simplifying this equation, we get y = x - 1.

Problem 5: Graphing a Line Graph the line that passes through the point (1, 2) and has a slope of 2.

Solution

To graph the line, we can start by plotting the point (1, 2). Then, we can use the slope to find another point on the line. Since the slope is 2, we can move 2 units up and 1 unit to the right to find another point on the line. Plotting this point and drawing a line through the two points, we get the graph of the line.

Problem 6: Writing in Point-Slope Form Write the equation of the line that passes through the point (3, 4) and has a slope of -2.

Solution

Using the point-slope form formula, we get y - 4 = -2(x - 3). Simplifying this equation, we get y = -2x + 10.

Problem 7: Finding the Equation Find the equation of the line that passes through the points (-2, 1) and (1, 3).

Solution

To find the equation, we can use the point-slope form formula. First, we need to find the slope using the formula m = (y2 - y1) / (x2 - x1). Plugging in the values, we get m = (3 - 1) / (1 - (-2)) = 1. Then, we can use the point-slope form formula to get y - 1 = 1(x - (-2)). Simplifying this equation, we get y = x + 3.

We hope these problems have helped you practice and solidify your understanding of point-slope form. Remember to use the point-slope form formula and to simplify your equations to get the final answer.