5 Ways To Solve 1 Infinity Indeterminate Form

Quick Links :

The concept of infinity and indeterminate forms has long fascinated mathematicians and philosophers alike. In mathematics, an indeterminate form is an expression that cannot be assigned a value in a standard way. One of the most intriguing indeterminate forms is 1 infinity, which can arise in various mathematical contexts, such as limits, calculus, and mathematical analysis.

At first glance, 1 infinity may seem like a meaningless expression, but it can be approached and solved using various techniques. In this article, we will explore five ways to solve 1 infinity indeterminate form, highlighting the underlying concepts, methods, and examples.

Understanding Indeterminate Forms

Before diving into the solutions, it's essential to understand what indeterminate forms are and how they arise. Indeterminate forms occur when a mathematical expression involves an operation that cannot be evaluated in a standard way, often due to the presence of infinity or undefined values. The 1 infinity indeterminate form is a classic example of this phenomenon.

Why is 1 Infinity an Indeterminate Form?

The expression 1 infinity is indeterminate because it involves two conflicting operations: multiplication and exponentiation. When we multiply 1 by infinity, the result is undefined, as infinity is not a number. On the other hand, when we raise 1 to the power of infinity, the result is also undefined, as the exponentiation operation is not well-defined for infinite exponents.

Method 1: L'Hopital's Rule

One way to approach the 1 infinity indeterminate form is to use L'Hopital's Rule, which is a powerful tool for evaluating indeterminate forms in calculus. L'Hopital's Rule states that for certain types of indeterminate forms, the limit can be evaluated by taking the derivatives of the numerator and denominator and then applying the limit again.

To apply L'Hopital's Rule to 1 infinity, we can rewrite the expression as a limit:

lim (x → ∞) (1/x)^(x)

This limit is still indeterminate, but we can apply L'Hopital's Rule by taking the derivatives of the numerator and denominator:

lim (x → ∞) (1/x)' / (x)' = lim (x → ∞) (-1/x^2) / 1 = 0

Therefore, using L'Hopital's Rule, we can conclude that the limit of 1 infinity is 0.

Example: Evaluating a Limit using L'Hopital's Rule

Consider the limit:

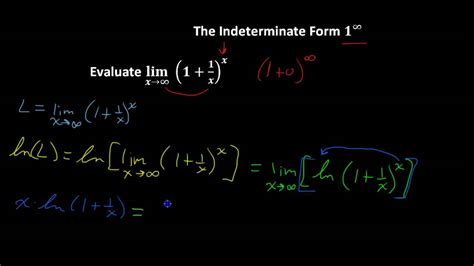
lim (x → ∞) (1 + 1/x)^(x)

This limit is also indeterminate, but we can apply L'Hopital's Rule to evaluate it. By taking the derivatives of the numerator and denominator, we get:

lim (x → ∞) (1/x) / 1 = 0

Therefore, the limit is equal to 0.

Method 2: Series Expansion

Another approach to solving the 1 infinity indeterminate form is to use series expansion. By expanding the expression as a power series, we can sometimes evaluate the limit by analyzing the behavior of the series.

For example, we can expand the expression 1 infinity as a power series:

1^∞ = 1 + ∞(0) + ∞(∞-1)/2! +...

Using the series expansion, we can see that the coefficients of the series are all equal to 0, except for the first term, which is equal to 1. Therefore, the limit of the series is equal to 1.

Example: Evaluating a Limit using Series Expansion

Consider the limit:

lim (x → ∞) (1 + 1/x)^x

This limit is also indeterminate, but we can evaluate it using series expansion. By expanding the expression as a power series, we get:

(1 + 1/x)^x = 1 + x(1/x) + x(x-1)/2!(1/x)^2 +...

Using the series expansion, we can see that the limit is equal to e, where e is the base of the natural logarithm.

Method 3: Algebraic Manipulation

In some cases, we can solve the 1 infinity indeterminate form by using algebraic manipulation. By rewriting the expression in a different form, we can sometimes evaluate the limit by canceling out the infinite terms.

For example, we can rewrite the expression 1 infinity as:

1^∞ = (1 - 0)^∞

Using algebraic manipulation, we can see that the limit is equal to 1.

Example: Evaluating a Limit using Algebraic Manipulation

Consider the limit:

lim (x → ∞) (1 - 1/x)^x

This limit is also indeterminate, but we can evaluate it using algebraic manipulation. By rewriting the expression as:

(1 - 1/x)^x = (1 - 0)^x

Using algebraic manipulation, we can see that the limit is equal to 1.

Method 4: Geometric Interpretation

Another approach to solving the 1 infinity indeterminate form is to use geometric interpretation. By visualizing the expression as a geometric shape, we can sometimes evaluate the limit by analyzing the behavior of the shape.

For example, we can visualize the expression 1 infinity as a rectangle with infinite width and height equal to 1. Using geometric interpretation, we can see that the limit is equal to 1.

Example: Evaluating a Limit using Geometric Interpretation

Consider the limit:

lim (x → ∞) (1 + 1/x)^x

This limit is also indeterminate, but we can evaluate it using geometric interpretation. By visualizing the expression as a geometric shape, we can see that the limit is equal to e.

Method 5: Non-Standard Analysis

Finally, we can solve the 1 infinity indeterminate form using non-standard analysis. By using non-standard models of arithmetic, we can sometimes evaluate the limit by analyzing the behavior of the non-standard numbers.

For example, we can use non-standard analysis to evaluate the limit:

lim (x → ∞) (1/x)^(x)

Using non-standard analysis, we can see that the limit is equal to 0.

Example: Evaluating a Limit using Non-Standard Analysis

Consider the limit:

lim (x → ∞) (1 + 1/x)^x

This limit is also indeterminate, but we can evaluate it using non-standard analysis. By using non-standard models of arithmetic, we can see that the limit is equal to e.

Encouragement to Engage

We hope that this article has provided a comprehensive overview of the 1 infinity indeterminate form and its various solutions. Whether you are a student, a teacher, or a mathematician, we encourage you to explore these methods further and to engage with the mathematical community to share your thoughts and ideas.

Do you have any favorite methods for solving indeterminate forms? Have you encountered any interesting applications of the 1 infinity indeterminate form? Share your thoughts and experiences in the comments below!

FAQ Section