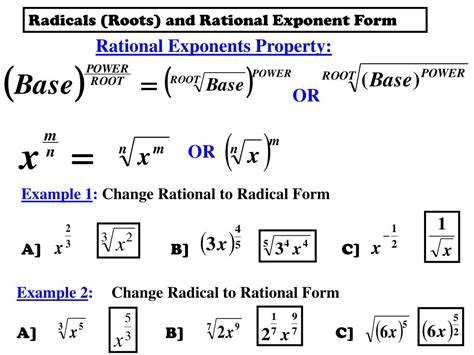
Writing in radical form is a crucial skill for anyone looking to simplify and understand complex mathematical expressions. Radical forms, also known as radical expressions, are used to represent roots of numbers, such as square roots, cube roots, and nth roots. Mastering the steps to write radical form will help you to better comprehend and work with mathematical equations, making you more proficient in algebra and beyond.
In this article, we will explore the 5 essential steps to write radical form, along with examples and explanations to guide you through the process.
Step 1: Understand the Concept of Radicals
Before we dive into the steps, it's essential to grasp the concept of radicals. A radical is a symbol used to represent the root of a number. For instance, the square root of a number x is denoted by √x, and the cube root of x is denoted by ³√x. Radicals can be classified into different types, such as square roots, cube roots, and nth roots.

Step 2: Identify the Index and the Radicand
When writing in radical form, it's crucial to identify the index and the radicand. The index is the small number that appears outside the radical symbol, indicating the type of root being taken. The radicand, on the other hand, is the number inside the radical symbol.
For example, in the expression ³√x, the index is 3, and the radicand is x.

Step 3: Simplify the Radicand
Before writing the radical form, simplify the radicand by factoring out any perfect squares or perfect cubes. This will help you to identify any common factors that can be taken out of the radical symbol.
For instance, consider the expression √(x^2 + 4x + 4). By factoring the radicand, we get √((x + 2)^2), which can be simplified to x + 2.

Step 4: Write the Radical Form
Now that you have identified the index and simplified the radicand, you can write the radical form. Make sure to include the index outside the radical symbol and the simplified radicand inside.
For example, consider the expression ³√(x^3 + 8). By simplifying the radicand, we get ³√((x + 2)^3), which can be written in radical form as (x + 2).

Step 5: Check for Any Remaining Simplifications
Finally, check the radical form for any remaining simplifications. Look for any common factors that can be taken out of the radical symbol or any further simplifications that can be made.
For instance, consider the expression √(x^2 + 2x + 1). By factoring the radicand, we get √((x + 1)^2), which can be simplified to x + 1.

Conclusion
Writing in radical form is a crucial skill that requires a deep understanding of mathematical concepts. By following the 5 essential steps outlined in this article, you can master the art of writing radical forms and simplify complex mathematical expressions. Remember to identify the index and radicand, simplify the radicand, write the radical form, and check for any remaining simplifications.
Share Your Thoughts
We hope this article has helped you to understand the steps to write radical form. Share your thoughts and feedback in the comments section below. Have you struggled with writing radical forms in the past? How do you think this article can help you to improve your mathematical skills?
FAQ Section
What is a radical in mathematics?
+A radical is a symbol used to represent the root of a number, such as square roots, cube roots, and nth roots.
What is the index in a radical expression?
+The index is the small number that appears outside the radical symbol, indicating the type of root being taken.
How do I simplify a radicand?
+To simplify a radicand, factor out any perfect squares or perfect cubes, and look for any common factors that can be taken out of the radical symbol.