Write An Ellipse In Standard Form Easily

Quick Links :

Understanding and working with ellipses in standard form can seem daunting, but with the right approach, it can be simplified and made accessible. In this article, we will delve into the world of ellipses, focusing on how to write an ellipse in standard form with ease.

Understanding Ellipses

An ellipse is a type of closed curve where the sum of the distances from any point on the curve to two fixed points (foci) is constant. Ellipses are used in various fields, including mathematics, physics, engineering, and architecture. Understanding ellipses is crucial in many applications, from designing satellite orbits to analyzing data in statistics.

Standard Form of an Ellipse

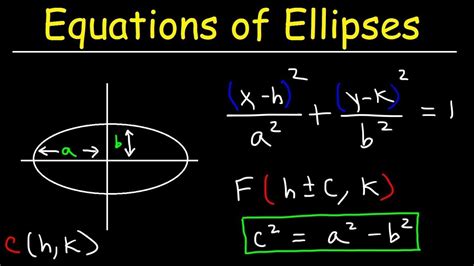
The standard form of an ellipse centered at (h,k) with semi-major axis 'a' and semi-minor axis 'b' is given by the equation:

(x - h)^2 / a^2 + (y - k)^2 / b^2 = 1

This equation represents a horizontal ellipse if a > b, and a vertical ellipse if a < b.

Key Components of the Standard Form

• Center (h, k): The point around which the ellipse is centered.
• Semi-major axis 'a': The longer radius of the ellipse.
• Semi-minor axis 'b': The shorter radius of the ellipse.

Steps to Write an Ellipse in Standard Form

1. Identify the center (h, k): Determine the coordinates of the center of the ellipse.
2. Determine the lengths of the semi-major axis 'a' and semi-minor axis 'b': Decide whether the ellipse is horizontal or vertical and identify the lengths of 'a' and 'b'.
3. Substitute the values into the standard form equation: Insert the values of h, k, a, and b into the equation (x - h)^2 / a^2 + (y - k)^2 / b^2 = 1.

Example: Writing an Ellipse in Standard Form

Suppose we have an ellipse with center (2, 3), semi-major axis 5, and semi-minor axis 3.

• Center (h, k) = (2, 3)
• Semi-major axis 'a' = 5
• Semi-minor axis 'b' = 3

Substituting these values into the standard form equation, we get:

(x - 2)^2 / 5^2 + (y - 3)^2 / 3^2 = 1

This is the standard form of the ellipse.

Practical Applications of Ellipses

Ellipses have numerous practical applications in various fields, including:

• Astronomy: Elliptical orbits of planets and satellites.
• Engineering: Designing elliptical tanks and pipelines.
• Architecture: Elliptical shapes in buildings and bridges.
• Statistics: Analyzing data using elliptical distributions.

Conclusion: Mastering Ellipses in Standard Form

Writing an ellipse in standard form may seem complex at first, but by understanding the key components and following the steps outlined in this article, you can master this skill with ease. Remember to practice and apply your knowledge to real-world scenarios to reinforce your understanding of ellipses.

What's your experience with ellipses? Share your thoughts and questions in the comments below!