Solve Quadratic Equations In Standard Form Made Easy

Quick Links :

Quadratic equations in standard form can be a daunting task for many students, but with the right approach, it can be made easy. In this article, we will break down the steps to solve quadratic equations in standard form and provide examples to illustrate the process.

Understanding Quadratic Equations in Standard Form

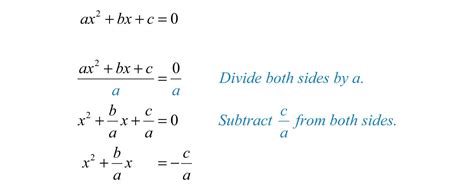
A quadratic equation in standard form is written as ax^2 + bx + c = 0, where a, b, and c are constants and x is the variable. The goal is to find the values of x that satisfy the equation. Quadratic equations can be solved using various methods, including factoring, the quadratic formula, and graphing.

Factoring Quadratic Equations

Factoring is a popular method for solving quadratic equations. It involves expressing the quadratic equation as a product of two binomials. The factored form of the equation is (x - r)(x - s) = 0, where r and s are the roots of the equation.

To factor a quadratic equation, we need to find two numbers whose product is c and whose sum is b. These numbers are the roots of the equation. For example, consider the quadratic equation x^2 + 5x + 6 = 0. We can factor this equation as (x + 3)(x + 2) = 0.

Steps to Factor Quadratic Equations

1. Write the quadratic equation in standard form.
2. Find two numbers whose product is c and whose sum is b.
3. Write the factored form of the equation as (x - r)(x - s) = 0.
4. Solve for x by setting each factor equal to zero.

The Quadratic Formula

The quadratic formula is a powerful tool for solving quadratic equations. It is given by x = (-b ± √(b^2 - 4ac)) / 2a. This formula can be used to solve any quadratic equation, regardless of whether it can be factored or not.

To use the quadratic formula, simply plug in the values of a, b, and c into the formula and simplify. For example, consider the quadratic equation x^2 + 4x + 4 = 0. We can use the quadratic formula to solve this equation as follows:

x = (-4 ± √(4^2 - 4(1)(4))) / 2(1) x = (-4 ± √(16 - 16)) / 2 x = (-4 ± √0) / 2 x = -4 / 2 x = -2

Steps to Use the Quadratic Formula

1. Write the quadratic equation in standard form.
2. Plug in the values of a, b, and c into the quadratic formula.
3. Simplify the expression and solve for x.

Graphing Quadratic Equations

Graphing is another method for solving quadratic equations. It involves plotting the graph of the quadratic equation and finding the x-intercepts. The x-intercepts are the values of x that satisfy the equation.

To graph a quadratic equation, we need to find the vertex and the axis of symmetry. The vertex is the point (h, k) where the graph reaches its maximum or minimum value. The axis of symmetry is the line x = h that divides the graph into two equal parts.

Steps to Graph Quadratic Equations

1. Write the quadratic equation in standard form.
2. Find the vertex and the axis of symmetry.
3. Plot the graph of the quadratic equation.
4. Find the x-intercepts and solve for x.

Conclusion

Solving quadratic equations in standard form can be made easy with the right approach. Factoring, the quadratic formula, and graphing are three popular methods for solving quadratic equations. By following the steps outlined in this article, you can master these methods and become proficient in solving quadratic equations.