Gauss's Law is a fundamental concept in physics, particularly in the study of electromagnetism. It is a powerful tool for calculating the electric field in various situations and has numerous applications in fields such as engineering, physics, and materials science. Gauss's Law is often expressed in integral form, but it can also be written in differential form, which provides a more detailed understanding of the relationship between electric charges and fields. In this article, we will delve into the world of Gauss's Law in differential form, exploring its definition, significance, and applications.

What is Gauss's Law in Differential Form?
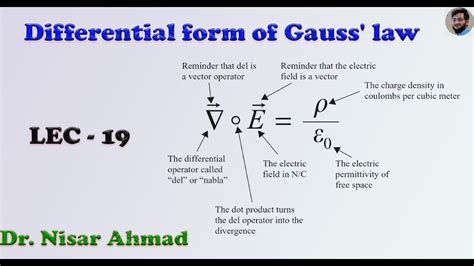
Gauss's Law in differential form is a mathematical statement that describes the relationship between the electric field and the distribution of electric charges. It is expressed as:
∇⋅E = ρ/ε₀
where E is the electric field, ρ is the charge density, and ε₀ is the electric constant (also known as the permittivity of free space). This equation states that the divergence of the electric field is proportional to the charge density.
Derivation of Gauss's Law in Differential Form
The differential form of Gauss's Law can be derived from the integral form, which is:
Φ = Q/ε₀
where Φ is the electric flux, Q is the total charge enclosed by a surface, and ε₀ is the electric constant. By applying the divergence theorem, we can rewrite the integral form as:
∇⋅E = ρ/ε₀
This derivation highlights the connection between the electric field and the charge density.

Significance of Gauss's Law in Differential Form
Gauss's Law in differential form provides a powerful tool for analyzing the behavior of electric fields in various situations. It is particularly useful for calculating the electric field in situations where the charge distribution is complex or non-uniform. The differential form also allows us to study the local behavior of the electric field, which is essential for understanding many phenomena in physics and engineering.
Applications of Gauss's Law in Differential Form
Gauss's Law in differential form has numerous applications in fields such as:
- Electromagnetism: Gauss's Law is used to calculate the electric field in various situations, including the behavior of charges in conductors and insulators.
- Materials Science: Gauss's Law is used to study the behavior of electric fields in materials, including the behavior of charges in semiconductors and dielectrics.
- Engineering: Gauss's Law is used in the design of electrical systems, including the design of capacitors, inductors, and transmission lines.

Solving Problems using Gauss's Law in Differential Form
Gauss's Law in differential form can be used to solve a variety of problems, including:
- Calculating the electric field in a uniform charge distribution
- Calculating the electric field in a non-uniform charge distribution
- Calculating the electric potential in a charge distribution
To solve these problems, we need to follow a few steps:
- Identify the charge distribution and the region of interest.
- Apply Gauss's Law in differential form to calculate the electric field.
- Use the electric field to calculate the electric potential.
Common Mistakes and Challenges**
When working with Gauss's Law in differential form, there are several common mistakes and challenges to be aware of:
- Misinterpreting the divergence of the electric field
- Misapplying the boundary conditions
- Failing to account for the symmetry of the charge distribution
By being aware of these challenges and taking the necessary precautions, we can ensure accurate and reliable results.

Conclusion and Future Directions
Gauss's Law in differential form is a powerful tool for analyzing the behavior of electric fields in various situations. Its applications range from electromagnetism to materials science and engineering. By understanding the derivation, significance, and applications of Gauss's Law in differential form, we can gain a deeper appreciation for the intricate relationships between electric charges and fields.
We hope this comprehensive guide has provided you with a solid foundation in Gauss's Law in differential form. Whether you're a student or a professional, we encourage you to continue exploring the fascinating world of electromagnetism and its many applications.
Share Your Thoughts!
We would love to hear your thoughts and feedback on this article. Have you used Gauss's Law in differential form in your work or studies? Do you have any questions or topics you'd like to discuss? Please share your comments below.
What is the main difference between Gauss's Law in integral and differential form?
+The main difference between Gauss's Law in integral and differential form is that the integral form describes the total electric flux through a closed surface, while the differential form describes the local behavior of the electric field.
How do I apply Gauss's Law in differential form to calculate the electric field?
+To apply Gauss's Law in differential form, you need to identify the charge distribution and the region of interest, and then use the equation ∇⋅E = ρ/ε₀ to calculate the electric field.
What are some common applications of Gauss's Law in differential form?
+Gauss's Law in differential form has numerous applications in fields such as electromagnetism, materials science, and engineering, including the design of electrical systems and the study of the behavior of electric fields in materials.