Exponential Form Of Sine Made Easy

Quick Links :

Unlocking the Secrets of Exponential Form of Sine

The exponential form of sine is a fundamental concept in mathematics, particularly in trigonometry and calculus. It is a way of expressing the sine function in terms of exponential functions, which can be incredibly useful for solving problems and understanding complex relationships. However, the exponential form of sine can be intimidating for many students, especially those who are new to advanced mathematics.

In this article, we will explore the exponential form of sine in detail, breaking down the concept into easy-to-understand pieces. We will also provide practical examples and tips to help you master this concept and apply it to real-world problems.

What is the Exponential Form of Sine?

The exponential form of sine is a way of expressing the sine function in terms of exponential functions. It is based on Euler's formula, which states that:

e^(ix) = cos(x) + i sin(x)

where e is the base of the natural logarithm, i is the imaginary unit, and x is the angle in radians.

By rearranging this formula, we can express the sine function in terms of exponential functions:

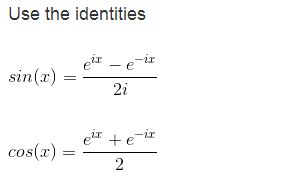
sin(x) = (e^(ix) - e^(-ix)) / 2i

This is the exponential form of sine.

Why is the Exponential Form of Sine Important?

The exponential form of sine is important for several reasons:

• Simplifies complex calculations: The exponential form of sine can be used to simplify complex calculations involving trigonometric functions. By expressing the sine function in terms of exponential functions, we can use the properties of exponential functions to simplify calculations.
• Provides a new perspective: The exponential form of sine provides a new perspective on the sine function, which can be useful for solving problems and understanding complex relationships.
• Used in advanced mathematics: The exponential form of sine is used in advanced mathematics, such as calculus and differential equations.

How to Use the Exponential Form of Sine

Using the exponential form of sine is relatively straightforward. Here are some steps to follow:

1. Express the sine function in exponential form: Use the formula sin(x) = (e^(ix) - e^(-ix)) / 2i to express the sine function in exponential form.
2. Simplify the expression: Use the properties of exponential functions to simplify the expression.
3. Apply the simplified expression: Apply the simplified expression to the problem you are trying to solve.

Examples of Using the Exponential Form of Sine

Here are some examples of using the exponential form of sine:

• Example 1: Find the value of sin(x) when x = π/2.
• Example 2: Simplify the expression sin(x) + sin(2x).
• Example 3: Solve the equation sin(x) = 1/2.

Tips for Mastering the Exponential Form of Sine

Here are some tips for mastering the exponential form of sine:

• Practice, practice, practice: Practice using the exponential form of sine to solve problems and simplify expressions.
• Use online resources: Use online resources, such as video tutorials and practice problems, to help you learn and master the exponential form of sine.
• Seek help when needed: Don't be afraid to seek help when you need it. Ask your teacher or tutor for help if you are struggling to understand the concept.

Conclusion

The exponential form of sine is a powerful tool for solving problems and understanding complex relationships. By mastering this concept, you can simplify complex calculations and gain a deeper understanding of trigonometry and calculus. Remember to practice regularly and seek help when needed to ensure that you become proficient in using the exponential form of sine.