5 Easy Ways To Calculate Parabola Equation

Quick Links :

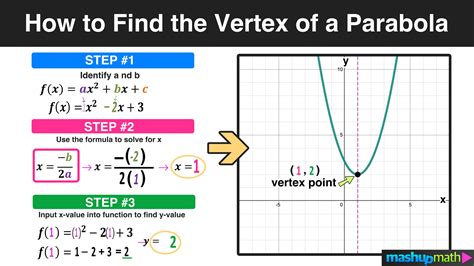
In mathematics, a parabola is a type of quadratic curve that is U-shaped and has a single turning point, known as the vertex. The parabola equation is a fundamental concept in algebra and geometry, and it is used to describe the relationship between the x and y coordinates of a point on the curve. In this article, we will explore five easy ways to calculate the parabola equation.

Understanding the Parabola Equation

The parabola equation is a quadratic equation in the form of y = ax^2 + bx + c, where a, b, and c are constants. The equation can also be written in vertex form as y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola.

Why is the Parabola Equation Important?

The parabola equation is an essential concept in mathematics and physics, as it is used to model various real-world phenomena, such as the trajectory of projectiles, the shape of mirrors and lenses, and the behavior of electrical circuits.

Method 1: Using the Vertex Form

One of the easiest ways to calculate the parabola equation is by using the vertex form. To do this, you need to know the coordinates of the vertex (h, k) and the value of the constant a. The equation is then written as y = a(x - h)^2 + k.

For example, if the vertex is at (2, 3) and a = 2, the equation would be y = 2(x - 2)^2 + 3.

Advantages of Using the Vertex Form

Using the vertex form has several advantages, including:

• It allows you to easily identify the vertex of the parabola.
• It makes it easy to determine the direction of the parabola (i.e., whether it opens upwards or downwards).
• It simplifies the process of graphing the parabola.

Method 2: Using the Standard Form

Another way to calculate the parabola equation is by using the standard form, which is y = ax^2 + bx + c. To use this method, you need to know the values of a, b, and c.

For example, if a = 2, b = 3, and c = 4, the equation would be y = 2x^2 + 3x + 4.

Advantages of Using the Standard Form

Using the standard form has several advantages, including:

• It allows you to easily identify the values of a, b, and c.
• It makes it easy to determine the x-intercepts of the parabola.
• It simplifies the process of finding the axis of symmetry.

Method 3: Using the Axis of Symmetry

The axis of symmetry is a vertical line that passes through the vertex of the parabola and is perpendicular to the x-axis. To use this method, you need to know the coordinates of the vertex and the value of the constant a.

For example, if the vertex is at (2, 3) and a = 2, the equation of the axis of symmetry would be x = 2.

Advantages of Using the Axis of Symmetry

Using the axis of symmetry has several advantages, including:

• It allows you to easily identify the vertex of the parabola.
• It makes it easy to determine the direction of the parabola.
• It simplifies the process of graphing the parabola.

Method 4: Using the X-Intercepts

The x-intercepts are the points where the parabola intersects the x-axis. To use this method, you need to know the values of the x-intercepts.

For example, if the x-intercepts are at (2, 0) and (4, 0), the equation would be y = a(x - 2)(x - 4).

Advantages of Using the X-Intercepts

Using the x-intercepts has several advantages, including:

• It allows you to easily identify the x-intercepts of the parabola.
• It makes it easy to determine the values of a, b, and c.
• It simplifies the process of graphing the parabola.

Method 5: Using the Slope-Intercept Form

The slope-intercept form is a linear equation in the form of y = mx + b, where m is the slope and b is the y-intercept. To use this method, you need to know the values of m and b.

For example, if m = 2 and b = 3, the equation would be y = 2x + 3.

Advantages of Using the Slope-Intercept Form

Using the slope-intercept form has several advantages, including:

• It allows you to easily identify the slope and y-intercept of the parabola.
• It makes it easy to determine the direction of the parabola.
• It simplifies the process of graphing the parabola.

Final Thoughts:

Calculating the parabola equation is an essential skill in mathematics and physics. By using one of the five methods outlined above, you can easily determine the equation of a parabola. Whether you use the vertex form, standard form, axis of symmetry, x-intercepts, or slope-intercept form, you can be sure that you will be able to accurately calculate the parabola equation.

If you have any questions or need further clarification on any of the methods outlined above, please don't hesitate to ask. We would love to hear from you and help you in any way we can.