Equation Of Ellipse In Standard Form Explained

Quick Links :

The equation of an ellipse in standard form is a fundamental concept in mathematics, particularly in algebra and geometry. Ellipses are closed curves that result from the intersection of a cone and a plane. They have numerous applications in various fields, including physics, engineering, and computer graphics. Understanding the equation of an ellipse in standard form is crucial for solving problems related to these fields.

The standard form of the equation of an ellipse is a concise way of representing the curve's properties, such as its center, major and minor axes, and vertices. It is essential to comprehend the components of this equation to work with ellipses effectively. In this article, we will delve into the details of the equation of an ellipse in standard form, explore its components, and provide examples to illustrate its application.

Components of the Equation of an Ellipse in Standard Form

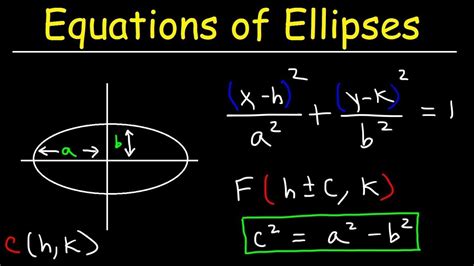
The standard form of the equation of an ellipse is given by:

(x - h)^2 / a^2 + (y - k)^2 / b^2 = 1

where:

• (h, k) represents the center of the ellipse
• a is the length of the semi-major axis (the longer radius)
• b is the length of the semi-minor axis (the shorter radius)

Understanding the Center of the Ellipse

The center of the ellipse, denoted by (h, k), is the midpoint of the major and minor axes. It is essential to identify the center of the ellipse, as it serves as a reference point for determining the orientation and size of the curve.

Major and Minor Axes of an Ellipse

The major axis of an ellipse is the longest diameter, which passes through the center of the ellipse. The semi-major axis, denoted by 'a', is half the length of the major axis. The minor axis is the shortest diameter, which also passes through the center of the ellipse. The semi-minor axis, denoted by 'b', is half the length of the minor axis.

Vertices of an Ellipse

The vertices of an ellipse are the points where the major axis intersects the curve. The distance from the center of the ellipse to either vertex is equal to the length of the semi-major axis 'a'.

Derivation of the Equation of an Ellipse in Standard Form

To derive the equation of an ellipse in standard form, we start with the definition of an ellipse as the set of all points for which the sum of the distances to two fixed points (foci) is constant. Let's consider an ellipse with center (h, k), semi-major axis 'a', and semi-minor axis 'b'. We can write the equation of the ellipse as:

(x - h)^2 / a^2 + (y - k)^2 / b^2 = 1

This equation can be derived by using the distance formula to express the sum of the distances from any point on the ellipse to the two foci.

Graphing an Ellipse in Standard Form

To graph an ellipse in standard form, we need to identify the center, major axis, and minor axis. We can then plot the vertices and sketch the curve. The equation of the ellipse provides us with the necessary information to graph the curve accurately.

Example: Graphing an Ellipse

Suppose we want to graph the ellipse with the equation:

(x - 2)^2 / 4 + (y + 1)^2 / 9 = 1

To graph this ellipse, we need to identify the center, major axis, and minor axis. The center of the ellipse is (2, -1), the semi-major axis is 3, and the semi-minor axis is 2. We can plot the vertices and sketch the curve.

Applications of the Equation of an Ellipse in Standard Form

The equation of an ellipse in standard form has numerous applications in various fields, including physics, engineering, and computer graphics. Some examples include:

• Orbits of celestial bodies
• Design of optical and acoustic systems
• Computer graphics and game development
• Architecture and construction

Conclusion

In conclusion, the equation of an ellipse in standard form is a fundamental concept in mathematics, with numerous applications in various fields. Understanding the components of this equation, including the center, major and minor axes, and vertices, is essential for working with ellipses effectively. By graphing an ellipse in standard form, we can visualize the curve and its properties. The equation of an ellipse in standard form provides a concise way of representing the curve's properties, making it a powerful tool for solving problems related to ellipses.