The world of mathematics can be both fascinating and intimidating, especially when it comes to solving complex equations. One of the most powerful tools in a math enthusiast's arsenal is the vertex form calculator. In this article, we will delve into the world of vertex form calculators, exploring their benefits, working mechanisms, and practical applications.
Understanding Vertex Form

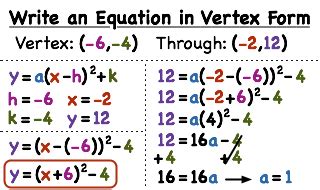
Before we dive into the calculator itself, let's first understand what vertex form is. In algebra, the vertex form of a quadratic function is a way of expressing the function in a more convenient and intuitive way. It is typically represented as f(x) = a(x-h)^2 + k, where (h,k) is the vertex of the parabola. This form makes it easier to identify the vertex, axis of symmetry, and direction of opening of the parabola.
Benefits of Vertex Form Calculator Tools
Using a vertex form calculator can greatly simplify the process of solving quadratic equations and graphing parabolas. Here are some benefits of using these calculators:
• Saves Time: Vertex form calculators can quickly convert a quadratic function from standard form to vertex form, saving you time and effort. • Reduces Errors: By automating the conversion process, these calculators minimize the risk of human error, ensuring accuracy and reliability. • Enhances Understanding: By providing a clear and concise representation of the parabola's vertex and axis of symmetry, vertex form calculators can help deepen your understanding of quadratic functions.
How Vertex Form Calculators Work

Vertex form calculators use a combination of mathematical algorithms and computational power to convert quadratic functions from standard form to vertex form. Here's a step-by-step explanation of how they work:
- Input: Enter the quadratic function in standard form (ax^2 + bx + c) into the calculator.
- Processing: The calculator uses a set of predefined algorithms to analyze the input function and identify the vertex (h,k).
- Conversion: The calculator converts the standard form function to vertex form (f(x) = a(x-h)^2 + k).
- Output: The calculator displays the vertex form function, along with the vertex coordinates (h,k).
Steps to Use a Vertex Form Calculator
Using a vertex form calculator is a straightforward process. Here are the steps:
- Choose a Calculator: Select a reliable and user-friendly vertex form calculator, either online or offline.
- Enter the Function: Input the quadratic function in standard form into the calculator.
- Click Calculate: Click the "Calculate" or "Convert" button to initiate the conversion process.
- Review the Results: Examine the output vertex form function and vertex coordinates.
Practical Applications of Vertex Form Calculators

Vertex form calculators have numerous practical applications in various fields, including:
• Algebra and Mathematics: Vertex form calculators are essential tools for students and professionals in algebra and mathematics, helping them solve quadratic equations and graph parabolas. • Physics and Engineering: Vertex form calculators can be used to model real-world phenomena, such as projectile motion and oscillations. • Data Analysis: Vertex form calculators can be applied in data analysis to identify patterns and trends in quadratic relationships.
Real-World Examples of Vertex Form Calculators
Here are a few real-world examples of vertex form calculators in action:
• Projectile Motion: A physics student uses a vertex form calculator to model the trajectory of a projectile, identifying the vertex (highest point) and axis of symmetry. • Economics: An economist uses a vertex form calculator to analyze the relationship between price and demand, identifying the vertex (optimal price) and axis of symmetry.
Conclusion
Vertex form calculators are powerful tools that can simplify the process of solving quadratic equations and graphing parabolas. By understanding how these calculators work and their practical applications, you can unlock the full potential of these tools and take your mathematical skills to the next level.

We hope this article has inspired you to explore the world of vertex form calculators and discover their many benefits. Share your thoughts and experiences with vertex form calculators in the comments below!
What is the vertex form of a quadratic function?
+The vertex form of a quadratic function is a way of expressing the function in the form f(x) = a(x-h)^2 + k, where (h,k) is the vertex of the parabola.
How do vertex form calculators work?
+Vertex form calculators use a combination of mathematical algorithms and computational power to convert quadratic functions from standard form to vertex form.
What are some practical applications of vertex form calculators?
+Vertex form calculators have numerous practical applications in various fields, including algebra and mathematics, physics and engineering, and data analysis.