5 Ways To Convert Intercept Form To Standard Form

Quick Links :

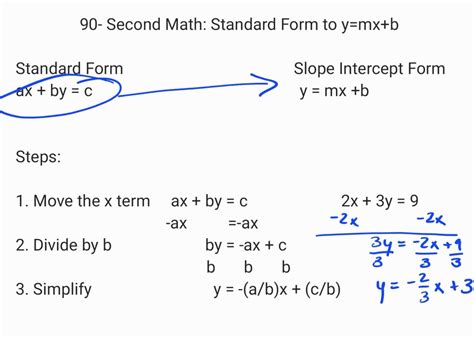
Converting equations from intercept form to standard form is a fundamental skill in algebra and mathematics. The intercept form of a linear equation is written as x/a + y/b = 1, where 'a' and 'b' are the x and y intercepts, respectively. In contrast, the standard form of a linear equation is written as Ax + By = C, where A, B, and C are constants. Mastering the conversion between these two forms can help you solve equations more efficiently and visualize the graph of the equation more effectively.

Understanding Intercept Form and Standard Form

Before we dive into the conversion methods, let's briefly review what intercept form and standard form represent in the context of linear equations.

• Intercept Form (x/a + y/b = 1): This form is useful for quickly identifying the x and y intercepts of a line. The x-intercept is the point where the line crosses the x-axis (where y=0), and the y-intercept is the point where the line crosses the y-axis (where x=0). The values of 'a' and 'b' directly represent these intercepts.

• Standard Form (Ax + By = C): The standard form is more versatile and is often used in solving systems of equations and graphing lines. A and B represent the coefficients of x and y, respectively, and C is the constant term. This form can also be used to find the x and y intercepts, though it requires a bit more calculation.

5 Methods to Convert Intercept Form to Standard Form

Method 1: Multiply Both Sides by the Least Common Multiple (LCM)

This method involves multiplying both sides of the equation by the least common multiple (LCM) of the denominators to clear the fractions. The LCM of 'a' and 'b' is often 'ab' itself if 'a' and 'b' have no common factors other than 1.

Example: Convert x/4 + y/3 = 1 to standard form.

• Multiply both sides by the LCM (12): 12*(x/4 + y/3) = 12*1
• Simplify: 3x + 4y = 12

Method 2: Use Algebraic Manipulation

Sometimes, simply rearranging the terms or multiplying by a strategic factor can make the conversion straightforward.

Example: Convert x/2 + y/-5 = 1 to standard form.

• Multiply both sides by 10 to clear the fractions: 10*(x/2 + y/-5) = 10*1
• Simplify: 5x - 2y = 10

Method 3: Identify the Coefficients and Constant Directly

For some equations, you can directly identify the coefficients of x and y and the constant term without extensive algebraic manipulation.

Example: Convert x/(-3) + y/2 = 1 to standard form.

• Multiply both sides by -6 to eliminate the fractions: -6*(x/(-3) + y/2) = -6*1
• Simplify: 2x - 3y = -6

Method 4: Use the x and y Intercepts

If you know the x and y intercepts, you can write the equation in standard form directly by using the intercepts as the coefficients of x and y and adjusting the signs and constant accordingly.

Example: If the x-intercept is -2 and the y-intercept is 3, the equation in standard form is x/-2 + y/3 = 1.

• Convert to standard form: 3x - 2y = -6

Method 5: Plotting Points and Finding the Equation

For a more visual approach, plot the x and y intercepts on a coordinate plane and find two additional points on the line. Use these points to find the equation of the line in standard form.

Example: Given intercepts (4,0) and (0,-3), plot two additional points on the line, such as (2,-1.5) and (0,-3).

• Use these points to write the equation in standard form.

Practical Applications and Conclusion

Converting linear equations from intercept form to standard form is a crucial skill that opens up various mathematical and real-world applications, from solving complex equations and inequalities to graphing lines and understanding the relationship between variables. By mastering these conversion methods, you'll become more adept at handling linear equations in different contexts, making you more versatile in problem-solving and analytical thinking.

What's Next?

Now that you've learned how to convert intercept form to standard form, practice applying these methods to various equations. Try solving systems of linear equations, graphing lines, and exploring how the standard form relates to real-world problems. Share your thoughts and questions about these methods in the comments below, and don't forget to share this article with anyone who might find it helpful!