805 Thousandths In Standard Form Explained

Quick Links :

Understanding the decimal system is crucial in mathematics, and being able to express numbers in standard form is a fundamental skill. In this article, we will delve into the world of decimals and explore how to express 805 thousandths in standard form.

What is Standard Form?

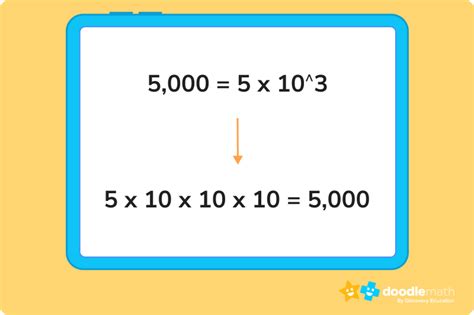
Standard form is a way of expressing numbers in a consistent and easily readable format. It is also known as scientific notation. In standard form, a number is written as a product of a number between 1 and 10, and a power of 10. For example, the number 456 can be written in standard form as 4.56 x 10^2.

Why is Standard Form Important?

Standard form is important because it allows us to easily compare and manipulate numbers. It is particularly useful when working with very large or very small numbers, as it helps to avoid errors and makes calculations more manageable. In addition, standard form is widely used in science, engineering, and mathematics, so it is an essential skill to have.

What are Thousandths?

Thousandths are a unit of measurement that represents one-thousandth of a whole. In decimal form, thousandths are represented by three digits after the decimal point. For example, 0.005 is five thousandths.

How to Express 805 Thousandths in Standard Form

To express 805 thousandths in standard form, we need to write it as a product of a number between 1 and 10, and a power of 10. Since 805 thousandths is equal to 0.805, we can write it in standard form as:

805 thousandths = 8.05 x 10^-1

This is because 8.05 is a number between 1 and 10, and 10^-1 is a power of 10 that represents the thousandths place.

Benefits of Expressing Numbers in Standard Form

Expressing numbers in standard form has several benefits. It makes numbers easier to read and compare, and it helps to avoid errors when performing calculations. Additionally, standard form is widely used in science, engineering, and mathematics, so it is an essential skill to have.

Real-World Applications of Standard Form

Standard form has many real-world applications. For example, it is used in science to express very large or very small numbers, such as the size of atoms or the distance to distant stars. It is also used in engineering to express measurements and calculations, such as the weight of materials or the stress on a bridge.

Common Mistakes When Expressing Numbers in Standard Form

There are several common mistakes that people make when expressing numbers in standard form. One of the most common mistakes is forgetting to include the power of 10. Another common mistake is using the wrong power of 10. For example, writing 805 thousandths as 8.05 x 10^2 instead of 8.05 x 10^-1.

Tips for Expressing Numbers in Standard Form

Here are some tips for expressing numbers in standard form:

• Always include the power of 10.
• Make sure the number is between 1 and 10.
• Use the correct power of 10.
• Practice, practice, practice!

Conclusion

In conclusion, expressing 805 thousandths in standard form is a simple process that requires attention to detail. By following the tips and guidelines outlined in this article, you can ensure that you are expressing numbers in standard form correctly. Remember to always include the power of 10, make sure the number is between 1 and 10, and use the correct power of 10.

Call to Action

We hope that this article has helped you to understand how to express 805 thousandths in standard form. If you have any questions or comments, please leave them in the section below. Don't forget to share this article with your friends and colleagues who may find it helpful.