Decimals In Expanded Form Made Easy For Students

Quick Links :

Understanding decimals in expanded form can be a challenging concept for many students. However, with a solid grasp of the basics and some practice, it can become a straightforward and essential math skill. In this article, we'll delve into the world of decimals in expanded form, making it easy for students to comprehend and apply this concept in their math studies.

What are Decimals in Expanded Form?

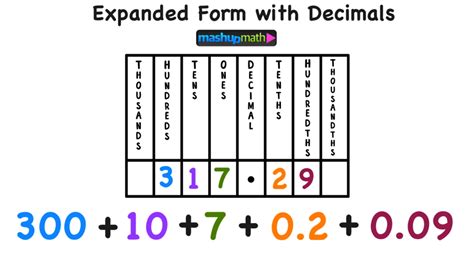
Decimals in expanded form represent a decimal number as the sum of its place values. This means that each digit in the decimal number is multiplied by its corresponding place value, and the results are added together. For example, the decimal number 4.25 can be written in expanded form as:

4 × 1 + 2 × 0.1 + 5 × 0.01

This representation helps students understand the relationship between the digits in a decimal number and their corresponding place values.

Why is it Important to Learn Decimals in Expanded Form?

Learning decimals in expanded form is crucial for several reasons:

• Deepens understanding of place value: Decimals in expanded form help students understand the concept of place value, which is essential for performing arithmetic operations with decimals.
• Improves mental math calculations: By representing decimals in expanded form, students can perform mental math calculations more efficiently and accurately.
• Enhances problem-solving skills: Decimals in expanded form enable students to approach problems in a more systematic and logical way, making it easier to solve complex math problems.

How to Write Decimals in Expanded Form

Writing decimals in expanded form involves multiplying each digit by its corresponding place value and adding the results together. Here's a step-by-step guide:

1. Identify the decimal number: Start by identifying the decimal number you want to write in expanded form.
2. Determine the place values: Determine the place values of each digit in the decimal number.
3. Multiply each digit by its place value: Multiply each digit by its corresponding place value.
4. Add the results together: Add the results together to get the expanded form of the decimal number.

Example: Write 6.37 in expanded form.

1. Identify the decimal number: 6.37
2. Determine the place values: 6 (ones), 3 (tenths), 7 (hundredths)
3. Multiply each digit by its place value: 6 × 1 + 3 × 0.1 + 7 × 0.01
4. Add the results together: 6 + 0.3 + 0.07 = 6.37

Real-World Applications of Decimals in Expanded Form

Decimals in expanded form have numerous real-world applications, including:

• Finance: Decimals in expanded form are used to calculate interest rates, investments, and expenses.
• Science: Decimals in expanded form are used to measure quantities such as temperature, pressure, and density.
• Engineering: Decimals in expanded form are used to design and build structures, machines, and electronic circuits.

Common Challenges and Solutions

Some common challenges students face when learning decimals in expanded form include:

• Difficulty in understanding place value: To overcome this, students can practice identifying the place values of digits in decimal numbers.
• Struggling with multiplication and addition: To overcome this, students can practice multiplying and adding decimals in expanded form.

Conclusion

Decimals in expanded form are an essential math concept that can seem daunting at first, but with practice and patience, it can become a valuable skill. By understanding the basics, practicing, and applying decimals in expanded form to real-world problems, students can build a strong foundation in math and develop problem-solving skills.

Practice and Reinforcement

To reinforce your understanding of decimals in expanded form, try the following practice exercises:

1. Write the following decimal numbers in expanded form:
   • 2.45
   • 7.93
   • 1.27
2. Calculate the sum of the following decimal numbers in expanded form:
   • 3.14 + 2.56
   • 1.23 + 4.56
   • 2.78 + 1.90

We encourage you to share your thoughts, ask questions, or provide feedback in the comments section below. Your input is valuable to us, and we look forward to hearing from you.