5 Steps To Convert To Vertex Form

Quick Links :

Converting equations to vertex form is an essential skill in algebra and pre-calculus. It allows us to easily identify the vertex of a parabola, which is a crucial point in understanding the graph's behavior. In this article, we will explore the steps to convert an equation to vertex form, along with practical examples and explanations.

Understanding Vertex Form

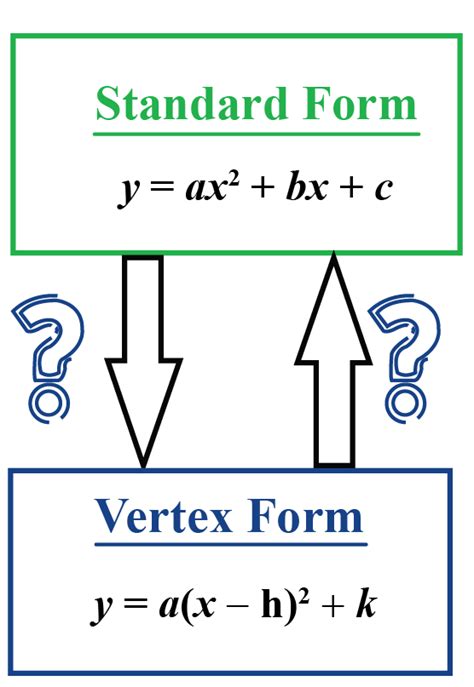
Before we dive into the steps, it's essential to understand what vertex form is. Vertex form is a way of expressing a quadratic equation in the form:

y = a(x - h)^2 + k

where (h, k) is the vertex of the parabola. This form is useful because it allows us to easily identify the vertex, which is the minimum or maximum point of the parabola.

Step 1: Write the Equation in Standard Form

The first step in converting an equation to vertex form is to write it in standard form. Standard form is a way of expressing a quadratic equation in the form:

ax^2 + bx + c = 0

where a, b, and c are constants. If the equation is already in standard form, you can move on to the next step. If not, you'll need to rewrite it in standard form.

Example:

Suppose we want to convert the equation:

x^2 + 5x - 6 = 0

to vertex form. The equation is already in standard form, so we can move on to the next step.

Step 2: Identify the Values of a, b, and c

In this step, we need to identify the values of a, b, and c in the standard form equation. In our example, the values are:

a = 1 b = 5 c = -6

These values will be used in the next steps to convert the equation to vertex form.

Step 3: Calculate the Value of h

To calculate the value of h, we use the formula:

h = -b / 2a

Plugging in the values we identified in step 2, we get:

h = -5 / (2*1) h = -5/2 h = -2.5

The value of h is the x-coordinate of the vertex.

Step 4: Calculate the Value of k

To calculate the value of k, we plug the value of h into the equation:

k = a(h)^2 + b(h) + c

Plugging in the values, we get:

k = 1(-2.5)^2 + 5(-2.5) - 6 k = 6.25 - 12.5 - 6 k = -12.25

The value of k is the y-coordinate of the vertex.

Step 5: Write the Equation in Vertex Form

Now that we have the values of h and k, we can write the equation in vertex form:

y = a(x - h)^2 + k y = 1(x + 2.5)^2 - 12.25

And that's it! We've successfully converted the equation to vertex form.

If you're still unsure about the process, don't worry. With practice, you'll become more comfortable converting equations to vertex form.

To get a better understanding of the concept, let's look at some more examples.

Example 1:

Convert the equation x^2 + 2x - 3 = 0 to vertex form.

Solution:

Using the steps outlined above, we get:

a = 1 b = 2 c = -3

h = -2 / (2*1) h = -1

k = 1(-1)^2 + 2(-1) - 3 k = 1 - 2 - 3 k = -4

y = 1(x + 1)^2 - 4

Example 2:

Convert the equation x^2 - 4x + 4 = 0 to vertex form.

Solution:

Using the steps outlined above, we get:

a = 1 b = -4 c = 4

h = 4 / (2*1) h = 2

k = 1(2)^2 - 4(2) + 4 k = 4 - 8 + 4 k = 0

y = 1(x - 2)^2

As you can see, converting equations to vertex form is a straightforward process. With practice, you'll become more comfortable with the steps, and you'll be able to convert equations quickly and easily.

Now that you've read this article, you might be wondering why converting equations to vertex form is important. Well, here are a few reasons:

• It allows you to easily identify the vertex of a parabola.
• It helps you understand the graph's behavior.
• It's a useful skill in algebra and pre-calculus.

If you have any questions or comments, please feel free to leave them below. We'd love to hear from you!