Converting repeating decimals to fractions can be a bit tricky, but don't worry, we've got you covered. In this article, we'll explore three ways to express 0.2 repeating as a fraction. Whether you're a math enthusiast or just looking for a quick solution, we'll break down the steps in a clear and concise manner.
Expressing 0.2 Repeating as a Fraction: Why Does it Matter?
Before we dive into the methods, let's understand why converting repeating decimals to fractions is important. In mathematics, fractions provide a more precise and concise way of representing numbers. They're essential in various mathematical operations, such as algebra, geometry, and calculus. Additionally, fractions can help simplify complex calculations and provide a deeper understanding of mathematical concepts.
Method 1: Using Algebraic Manipulation

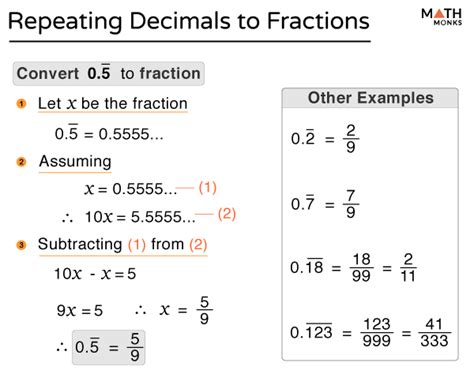
Let's start with the first method, which involves using algebraic manipulation. We'll represent 0.2 repeating as 'x' and subtract it from 10x to eliminate the repeating decimal.
x = 0.222222... 10x = 2.222222...
Now, let's subtract the first equation from the second:
10x - x = 2.222222... - 0.222222... 9x = 2 x = 2/9
So, 0.2 repeating can be expressed as the fraction 2/9.
Method 2: Using Geometric Series

The second method involves using geometric series to convert the repeating decimal to a fraction. We can represent 0.2 repeating as an infinite geometric series:
0.2 + 0.02 + 0.002 +...
The first term is 0.2, and the common ratio is 0.1. We can use the formula for the sum of an infinite geometric series to find the fraction:
S = a / (1 - r) = 0.2 / (1 - 0.1) = 0.2 / 0.9 = 2/9
Again, we've found that 0.2 repeating can be expressed as the fraction 2/9.
Method 3: Using Long Division

The third method involves using long division to convert the repeating decimal to a fraction. We'll divide 0.2 by 1 to get the repeating decimal:
0.222222...
Now, let's perform long division:
1 | 0.222222...
- 2
0.022222...
- 0.2
0.022222...
- 0.02
0.002222...
- 0.002
0.000222...
...
As we can see, the decimal repeats indefinitely. We can stop here and express the result as a fraction:
0.2 repeating = 2/9
All three methods yield the same result: 0.2 repeating can be expressed as the fraction 2/9.
Takeaways and Final Thoughts
Converting repeating decimals to fractions can be a bit challenging, but with the right techniques, it's achievable. We've explored three methods to express 0.2 repeating as a fraction: algebraic manipulation, geometric series, and long division. Each method provides a unique perspective on the problem, and understanding them can help you tackle more complex mathematical concepts.
Now, we'd love to hear from you! Do you have any favorite methods for converting repeating decimals to fractions? Share your thoughts in the comments below.
What is a repeating decimal?
+A repeating decimal is a decimal representation of a number that has a repeating pattern of digits after the decimal point.
Why is it important to convert repeating decimals to fractions?
+Converting repeating decimals to fractions provides a more precise and concise way of representing numbers, which is essential in various mathematical operations.
Can I use other methods to convert repeating decimals to fractions?
+Yes, there are other methods available, such as using calculators or computer programs. However, the methods presented in this article provide a more in-depth understanding of the mathematical concepts involved.