5 Steps To Convert To Phasor Form

Quick Links :

Converting to phasor form is an essential process in the analysis of AC circuits, allowing for the simplification of complex calculations and the representation of circuit behavior in a more intuitive manner. This process involves transforming sinusoidal signals into complex numbers, known as phasors, which capture both the magnitude and phase angle of the signals. Understanding how to convert to phasor form is crucial for electrical engineers, technicians, and anyone involved in the study or application of AC circuit analysis.

The importance of converting to phasor form lies in its ability to significantly simplify the analysis of AC circuits. By representing voltages, currents, and impedances as phasors, engineers can apply complex number arithmetic to solve circuit problems more efficiently. This is particularly useful in power systems, where phasors enable the calculation of power flows and voltages under various load conditions. Moreover, the phasor representation is the foundation for more advanced topics in electrical engineering, such as power system stability analysis and control system design.

In practical terms, converting to phasor form involves a series of systematic steps that ensure accuracy and ease of calculation. These steps are universally applicable to all types of AC circuits, making them a fundamental skill for anyone engaged in circuit analysis. Whether you're a seasoned engineer or just beginning your studies, mastering the conversion to phasor form is an essential part of your toolkit.

Step 1: Identify the Sinusoidal Signal

The first step in converting to phasor form is to identify the sinusoidal signal of interest. This could be a voltage or current in an AC circuit, represented by a sinusoidal waveform. It's crucial to note the peak value (amplitude) and the phase angle of the signal. The phase angle is the angular displacement of the signal from a reference point, usually the positive x-axis. In mathematical terms, a sinusoidal signal can be represented as (v(t) = V_m \cos(\omega t + \theta)) or (i(t) = I_m \cos(\omega t + \theta)), where (V_m) or (I_m) is the peak amplitude, (\omega) is the angular frequency, (t) is time, and (\theta) is the phase angle.

Notation and Units

• (V_m) or (I_m): Peak amplitude of voltage or current.
• (\omega): Angular frequency, in radians per second.
• (t): Time, in seconds.
• (\theta): Phase angle, in radians.

Step 2: Convert to Complex Number Form

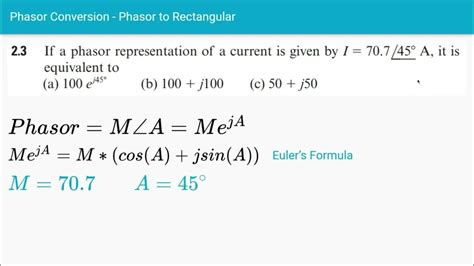
Once you have identified and represented your signal, the next step is to convert it into a complex number form. This involves expressing the signal in terms of its real and imaginary components, which correspond to the cosine and sine components of the original sinusoidal signal. The complex number form can be represented as (V = V_m e^{j\theta}) or (I = I_m e^{j\theta}), where (j) is the imaginary unit (the square root of -1). This form captures both the magnitude and phase of the signal in a single mathematical entity.

Complex Number Representation

• (V) or (I): Complex representation of voltage or current.
• (V_m) or (I_m): Peak amplitude.
• (e^{j\theta}): Complex exponential representing phase angle (\theta).

Step 3: Determine the Phasor Form

Now that you have the complex number form of your signal, determining the phasor form involves dividing the complex number by (e^{j\omega t}), which is essentially removing the time-varying part of the sinusoidal signal. This leaves you with a phasor that represents the signal's magnitude and phase angle in a way that is independent of time. The phasor form can be represented as (\mathbf{V} = V_m \angle \theta) or (\mathbf{I} = I_m \angle \theta), where (\angle) denotes the angle in degrees.

Phasor Notation

• (\mathbf{V}) or (\mathbf{I}): Phasor representation of voltage or current.
• (V_m) or (I_m): Peak amplitude.
• (\angle \theta): Phase angle in degrees.

Step 4: Calculate Impedance

After converting voltages and currents to phasor form, the next step involves calculating the impedance of the circuit. Impedance ((\mathbf{Z})) is the ratio of voltage to current, or (\mathbf{Z} = \mathbf{V} / \mathbf{I}). In the context of AC circuits, impedance is a complex quantity that includes both resistance and reactance. The calculation of impedance is crucial because it allows for the analysis of how voltages and currents interact within the circuit.

Impedance Calculation

• (\mathbf{Z}): Impedance of the circuit.
• (\mathbf{V}): Phasor voltage.
• (\mathbf{I}): Phasor current.

Step 5: Apply Phasor Analysis

The final step in the process is to apply phasor analysis to solve circuit problems. This involves using the phasor forms of voltages, currents, and impedances to analyze circuit behavior under various conditions. By performing arithmetic operations (addition, subtraction, multiplication, and division) on phasors, you can solve for unknown quantities within the circuit, such as voltages across specific components or currents through branches. The application of phasor analysis enables the efficient solution of complex AC circuit problems, providing insights into circuit performance and behavior.

Phasor Analysis Applications

• Circuit simplification.
• Voltage and current calculations.
• Impedance calculations.
• Power calculations.

By following these five steps, you can convert sinusoidal signals to phasor form, facilitating the analysis of AC circuits. This process is foundational in electrical engineering and has widespread applications in the design, analysis, and operation of electrical power systems. Remember, the key to mastering phasor analysis lies in understanding the mathematical transformations and applying them systematically to solve circuit problems.