Convert Factored Form To Standard Form Made Easy

Quick Links :

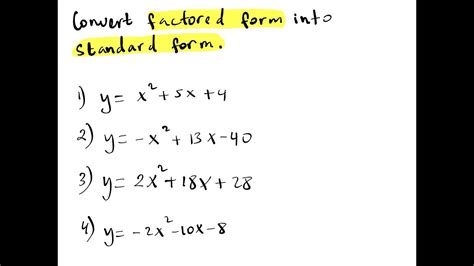
Converting factored form to standard form is a crucial skill in algebra, and it's easier than you think. In this article, we'll break down the process step by step, providing you with a comprehensive guide on how to convert factored form to standard form with ease.

Understanding Factored Form and Standard Form

Before we dive into the conversion process, it's essential to understand the difference between factored form and standard form. Factored form is a way of expressing a quadratic equation in the form of (x - a)(x - b) = 0, where 'a' and 'b' are the roots of the equation. On the other hand, standard form is a way of expressing a quadratic equation in the form of ax^2 + bx + c = 0, where 'a', 'b', and 'c' are coefficients.

The Importance of Converting Factored Form to Standard Form

Converting factored form to standard form is crucial in algebra, as it allows you to solve quadratic equations and graph quadratic functions. By converting factored form to standard form, you can identify the roots of the equation, determine the axis of symmetry, and graph the function with ease.

The Step-by-Step Process of Converting Factored Form to Standard Form

Now that we've covered the basics, let's dive into the step-by-step process of converting factored form to standard form.

Step 1: Identify the Roots of the Equation

To convert factored form to standard form, you need to identify the roots of the equation. The roots of the equation are the values of 'x' that make the equation true. In factored form, the roots are the values of 'a' and 'b' in the expression (x - a)(x - b) = 0.

Step 2: Multiply the Binomials

Once you've identified the roots of the equation, you need to multiply the binomials. To do this, you need to follow the FOIL method, which stands for "First, Outer, Inner, Last." This method involves multiplying the first terms of each binomial, then the outer terms, then the inner terms, and finally the last terms.

Step 3: Combine Like Terms

After multiplying the binomials, you need to combine like terms. This involves adding or subtracting the coefficients of the like terms.

Step 4: Write the Equation in Standard Form

Finally, you need to write the equation in standard form. This involves arranging the terms in the order of ax^2 + bx + c = 0.

Examples of Converting Factored Form to Standard Form

Here are a few examples of converting factored form to standard form:

Example 1:

Factored Form: (x - 2)(x + 3) = 0

Standard Form: x^2 + x - 6 = 0

Example 2:

Factored Form: (x + 1)(x - 4) = 0

Standard Form: x^2 - 3x - 4 = 0

Common Mistakes to Avoid When Converting Factored Form to Standard Form

When converting factored form to standard form, there are a few common mistakes to avoid:

• Forgetting to multiply the binomials: This is the most common mistake when converting factored form to standard form. Make sure to follow the FOIL method to multiply the binomials.
• Not combining like terms: After multiplying the binomials, make sure to combine like terms. This will ensure that your equation is in standard form.

Conclusion: Making Converting Factored Form to Standard Form Easy

Converting factored form to standard form is a crucial skill in algebra, and it's easier than you think. By following the step-by-step process outlined in this article, you can convert factored form to standard form with ease. Remember to identify the roots of the equation, multiply the binomials, combine like terms, and write the equation in standard form. With practice, you'll become a pro at converting factored form to standard form in no time!

We hope this article has been helpful in making converting factored form to standard form easy. If you have any questions or comments, please feel free to share them below.