4 Ways To Find Vertex Form With A Calculator

Quick Links :

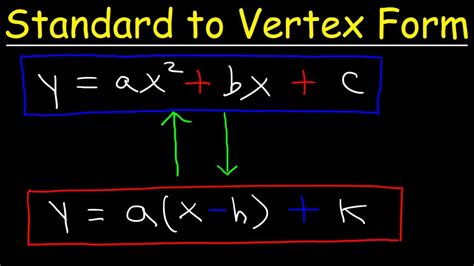
As a student of mathematics, you're likely no stranger to the world of quadratic equations and their various forms. One of the most useful forms of a quadratic equation is the vertex form, which provides valuable information about the parabola's vertex and axis of symmetry. While finding the vertex form manually can be a tedious and error-prone process, a calculator can greatly simplify the task. In this article, we'll explore four ways to find vertex form with a calculator, using both graphing and non-graphing calculators.

Method 1: Using a Graphing Calculator's Equation Solver

A graphing calculator is a powerful tool for solving equations, including quadratic equations. To find the vertex form using a graphing calculator's equation solver, follow these steps:

1. Enter the quadratic equation in the equation solver. For example, if you want to find the vertex form of the equation x^2 + 5x + 6 = 0, enter it as x^2 + 5x + 6 = 0.
2. Press the "Solve" button to find the solutions to the equation. The calculator will display the solutions in the form x = a ± √(b).
3. Take the solution with the positive sign (a + √(b)) and rewrite it in the form x = h ± √(k), where h is the x-coordinate of the vertex and k is the distance from the vertex to the focus.
4. The vertex form of the equation is now y = a(x - h)^2 + k.

Using this method, you can quickly find the vertex form of a quadratic equation using a graphing calculator.

Method 2: Using a Graphing Calculator's Vertex Formula

Another way to find the vertex form using a graphing calculator is to use the vertex formula. The vertex formula is given by:

x = (-b ± √(b^2 - 4ac)) / 2a

To use this formula on a graphing calculator, follow these steps:

1. Enter the values of a, b, and c into the calculator. For example, if you want to find the vertex form of the equation x^2 + 5x + 6 = 0, enter a = 1, b = 5, and c = 6.
2. Press the "Calc" button to calculate the value of x.
3. Take the value of x and rewrite it in the form x = h ± √(k), where h is the x-coordinate of the vertex and k is the distance from the vertex to the focus.
4. The vertex form of the equation is now y = a(x - h)^2 + k.

Using the vertex formula on a graphing calculator is a quick and easy way to find the vertex form of a quadratic equation.

Method 3: Using a Non-Graphing Calculator's Quadratic Formula

If you don't have a graphing calculator, you can still find the vertex form using a non-graphing calculator's quadratic formula. The quadratic formula is given by:

x = (-b ± √(b^2 - 4ac)) / 2a

To use this formula on a non-graphing calculator, follow these steps:

1. Enter the values of a, b, and c into the calculator. For example, if you want to find the vertex form of the equation x^2 + 5x + 6 = 0, enter a = 1, b = 5, and c = 6.
2. Press the "Calc" button to calculate the value of x.
3. Take the value of x and rewrite it in the form x = h ± √(k), where h is the x-coordinate of the vertex and k is the distance from the vertex to the focus.
4. The vertex form of the equation is now y = a(x - h)^2 + k.

Using the quadratic formula on a non-graphing calculator is a bit more tedious than using a graphing calculator, but it still gets the job done.

Method 4: Using a Calculator's Conic Section Formula

Some calculators have a built-in conic section formula that can be used to find the vertex form of a quadratic equation. The conic section formula is given by:

(x - h)^2 / a^2 + (y - k)^2 / b^2 = 1

To use this formula on a calculator, follow these steps:

1. Enter the values of a, b, and c into the calculator. For example, if you want to find the vertex form of the equation x^2 + 5x + 6 = 0, enter a = 1, b = 5, and c = 6.
2. Press the "Calc" button to calculate the values of h and k.
3. Take the values of h and k and rewrite the equation in the form y = a(x - h)^2 + k.
4. The vertex form of the equation is now complete.

Using the conic section formula on a calculator is a quick and easy way to find the vertex form of a quadratic equation.

In conclusion, finding the vertex form of a quadratic equation with a calculator is a straightforward process that can be accomplished in several ways. Whether you're using a graphing calculator or a non-graphing calculator, these four methods can help you find the vertex form quickly and accurately.

Benefits of Finding Vertex Form with a Calculator

There are several benefits to finding the vertex form of a quadratic equation with a calculator. Some of the benefits include:

• Increased accuracy: Using a calculator to find the vertex form eliminates the possibility of human error, ensuring that your answers are accurate and reliable.
• Faster calculations: Calculators can perform calculations much faster than humans, saving you time and effort when working with quadratic equations.
• Improved understanding: By using a calculator to find the vertex form, you can gain a deeper understanding of the underlying mathematics and how the formula works.

Overall, finding the vertex form of a quadratic equation with a calculator is a valuable skill that can help you succeed in mathematics and other STEM fields.

Common Applications of Vertex Form

The vertex form of a quadratic equation has many practical applications in science, engineering, and finance. Some common applications include:

• Projectile motion: The vertex form can be used to model the trajectory of projectiles under the influence of gravity.
• Optimization problems: The vertex form can be used to find the maximum or minimum value of a quadratic function.
• Electrical engineering: The vertex form can be used to analyze and design electronic circuits.

Overall, the vertex form is a powerful tool that can be used to solve a wide range of problems in various fields.

Conclusion

Finding the vertex form of a quadratic equation with a calculator is a valuable skill that can help you succeed in mathematics and other STEM fields. By using one of the four methods outlined in this article, you can quickly and accurately find the vertex form of a quadratic equation. Whether you're a student or a professional, mastering the vertex form can help you solve a wide range of problems and achieve your goals.