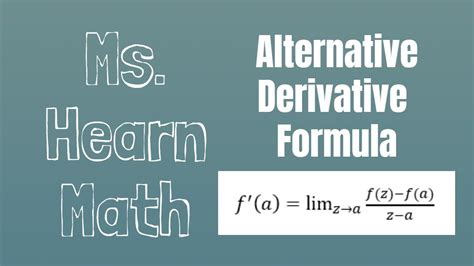
Alternate form derivatives are a fundamental concept in calculus, and understanding them can be a game-changer for students and professionals alike. In this article, we will delve into the world of alternate form derivatives, exploring five ways to grasp this concept.
What are Alternate Form Derivatives?
Alternate form derivatives, also known as alternate derivative forms or alternative derivative notations, refer to different ways of expressing the derivative of a function. These forms provide alternative perspectives on the derivative, making it easier to apply and interpret in various mathematical and real-world contexts.
1. Understanding Alternate Form Derivatives through Notation

One way to understand alternate form derivatives is by familiarizing yourself with different notations. The most common notations for derivatives are:
- Lagrange's notation: f'(x)
- Leibniz's notation: d/dx f(x) or (df/dx)(x)
- Newton's notation: ẋ or ḟ(x)
Each notation has its strengths and weaknesses, and being able to switch between them can help you better understand the concept of alternate form derivatives.
2. Visualizing Alternate Form Derivatives with Graphs

Visualizing alternate form derivatives can be a powerful way to understand this concept. By graphing functions and their derivatives, you can see how different forms of the derivative can provide distinct insights into the behavior of the function.
For example, graphing a function and its derivative in Leibniz's notation can help you understand how the derivative represents the rate of change of the function.
3. Exploring Alternate Form Derivatives through Examples

Working through examples is an excellent way to solidify your understanding of alternate form derivatives. By applying different forms of the derivative to various functions, you can develop a deeper understanding of how they work and when to use them.
For instance, finding the derivative of a polynomial function using Lagrange's notation and then converting it to Leibniz's notation can help you appreciate the flexibility of alternate form derivatives.
4. Understanding Alternate Form Derivatives through Calculus Theorems

Calculus theorems, such as the Power Rule and the Product Rule, provide a solid foundation for understanding alternate form derivatives. By mastering these theorems, you can develop a deeper understanding of how different forms of the derivative can be applied to various functions.
For example, using the Power Rule to find the derivative of a function and then expressing it in Newton's notation can help you see the connection between the derivative and the original function.
5. Practicing Alternate Form Derivatives with Real-World Applications

Finally, practicing alternate form derivatives with real-world applications can help you appreciate their relevance and importance. By working on problems that involve physics, engineering, economics, or other fields, you can see how different forms of the derivative can be used to model and analyze real-world phenomena.
For instance, using Leibniz's notation to find the derivative of a function that represents the velocity of an object can help you understand how the derivative can be used to predict the object's motion.
What is the difference between Lagrange's notation and Leibniz's notation?
+Lagrange's notation uses a prime symbol (f') to denote the derivative, while Leibniz's notation uses a fraction (d/dx f(x)) to represent the derivative.
How do I choose the right notation for a problem?
+The choice of notation depends on the specific problem and the context in which it is being used. For example, Leibniz's notation is often used in physics and engineering, while Lagrange's notation is commonly used in mathematics and computer science.
Can I use multiple notations in the same problem?
+Yes, it is often helpful to use multiple notations in the same problem, as each notation can provide a unique perspective on the derivative.
In conclusion, understanding alternate form derivatives requires a combination of notation, visualization, examples, calculus theorems, and real-world applications. By mastering these five ways, you can develop a deeper appreciation for the power and flexibility of alternate form derivatives. So, take the time to practice, explore, and apply these concepts, and you will become proficient in using alternate form derivatives in no time!