Unlocking a World of Possibilities: Simplifying 9 to 36

Mastering math concepts, particularly those related to fractions and proportions, is a fundamental skill that opens doors to a deeper understanding of various mathematical principles. The 9 to 36 simplification process, while seemingly complex at first glance, can be broken down into easy-to-follow steps that make the entire process accessible to learners of all levels.
Step 1: Understand the Basics
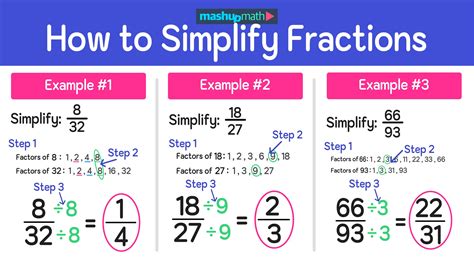
To simplify 9 to 36, it's crucial to grasp the underlying concept of equivalent fractions. An equivalent fraction is obtained by multiplying or dividing both the numerator and the denominator of a fraction by the same non-zero number. This operation does not change the value of the fraction, allowing for various representations of the same ratio.Breaking Down the Simplification Process

Step 2: Identify Common Factors
To simplify the fraction 9/36, the next step involves identifying any common factors between the numerator (9) and the denominator (36). A common factor is a number that divides both numbers evenly without leaving a remainder.
Step 3: Determine the Greatest Common Divisor (GCD)
After identifying the common factors, the next step is to find the greatest among them, known as the Greatest Common Divisor (GCD). The GCD of 9 and 36 is 9.
Step 4: Divide Both the Numerator and Denominator by the GCD
Once the GCD is identified, divide both the numerator and the denominator by this number to simplify the fraction. Simplifying 9/36 using the GCD:
- Numerator (9) ÷ GCD (9) = 1
- Denominator (36) ÷ GCD (9) = 4
Thus, the simplified form of 9/36 is 1/4.
Practical Applications of Simplification

The process of simplifying fractions is not merely a theoretical exercise; it has numerous practical applications in various fields, including science, engineering, economics, and everyday life. Understanding how to simplify fractions allows for the efficient comparison of ratios, the calculation of proportions, and the solution of problems involving scaling and measurement.
Real-World Example: Cooking and Recipes
In cooking, recipes often require adjustments in ingredient quantities. Simplifying fractions makes it easier to scale recipes up or down. For instance, if a recipe serving four people requires 9/36 of a teaspoon of a spice, simplifying this fraction to 1/4 teaspoon makes it clear that for a single serving, one would need a quarter of a teaspoon of the spice.Mastery Through Practice

Like any skill, mastering the art of simplifying fractions requires practice. The more one practices identifying common factors, determining GCDs, and simplifying fractions, the more intuitive and straightforward the process becomes.
Tips for Effective Practice
- Start with simple fractions and gradually move to more complex ones. - Use real-world examples to make the practice more engaging and relevant. - Apply the simplification process to solve everyday problems or puzzles.Embracing the World of Fractions

Understanding fractions and mastering the skill of simplification open up a broader appreciation for mathematical concepts. From the intricate patterns in nature to the advanced calculations in technology and science, fractions play a pivotal role.
Expanding Your Knowledge
Beyond simplification, exploring other operations with fractions, such as addition, subtraction, multiplication, and division, can further deepen your understanding of mathematics. Each operation presents its challenges and insights, offering a comprehensive grasp of fractions and their role in problem-solving.Conclusion and Next Steps

Simplifying fractions is a foundational skill that, once mastered, can lead to a greater understanding and appreciation of mathematics. By following the steps outlined and practicing with various fractions, individuals can overcome initial difficulties and unlock a world of possibilities.
Come Join the Conversation
We invite you to share your thoughts on simplifying fractions and how this skill has helped you in your academic or professional journey. What challenges did you face, and how did you overcome them? Your experiences and insights can inspire and guide others seeking to master this fundamental math concept.What is the purpose of simplifying fractions?
+Simplifying fractions makes it easier to compare, add, subtract, multiply, and divide them, and it has numerous practical applications in various fields.
How do I simplify a fraction?
+To simplify a fraction, find the greatest common divisor (GCD) of the numerator and denominator, then divide both by the GCD.
What are some practical applications of simplifying fractions?
+Simplifying fractions is useful in cooking, science, engineering, economics, and everyday life for tasks like scaling recipes, calculating proportions, and solving problems involving measurement.