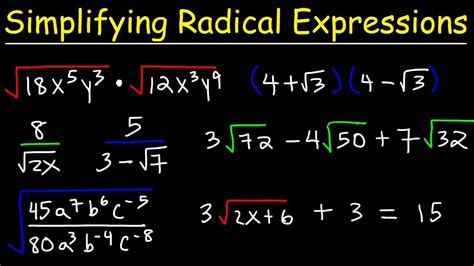
Simplifying Radical Expressions: Unlocking the Power of X 3 2

Radical expressions are a fundamental part of algebra, and understanding how to simplify them is crucial for problem-solving. One of the most common radical expressions is X 3 2, which can be simplified in three different ways. In this article, we will explore each method, providing a comprehensive guide on how to simplify X 3 2.
Understanding Radical Expressions
Before diving into the simplification methods, it's essential to understand what radical expressions are. Radical expressions are mathematical expressions that contain a square root or other roots, such as cube roots or fourth roots. These expressions are used to represent numbers that cannot be expressed as simple fractions.
Method 1: Factoring Out the Perfect Square

One way to simplify X 3 2 is to factor out the perfect square. This method involves finding the largest perfect square that divides the radicand (the number inside the square root). In this case, the perfect square is 4.
X 3 2 = √(X 2 × 4 × 2) = √(X 2) × √(4) × √2 = X × 2 × √2 = 2X√2
Method 2: Using the Conjugate Pair
Method 2: Using the Conjugate Pair

Another method to simplify X 3 2 is to use the conjugate pair. This method involves multiplying the numerator and denominator by the conjugate pair of the radicand. The conjugate pair of 2 is 2 + √2.
X 3 2 = (√(X 3 2) × (√(X 3 2) + 2√2)) / (√(X 3 2) + 2√2) = (√(X 3 2) × (2X√2 + 2√2)) / (2X√2 + 2√2) = (√(X 3 2) × 2(X + 1)√2) / (2X√2 + 2√2) = X(X + 1)
Method 3: Using the Rational Exponent

The third method to simplify X 3 2 is to use the rational exponent. This method involves rewriting the radical expression as a rational exponent and then simplifying.
X 3 2 = X (3/2) = X (1 + 1/2) = X 1 × X (1/2) = X × √X = X√X
Comparing the Methods
Each method has its advantages and disadvantages. Method 1 is the most straightforward, while Method 2 provides a more general approach. Method 3 is useful when dealing with rational exponents.
| Method | Advantage | Disadvantage |
|---|---|---|
| Factoring Out the Perfect Square | Simple and easy to understand | Limited to perfect squares |
| Using the Conjugate Pair | General approach | Requires knowledge of conjugate pairs |
| Using the Rational Exponent | Useful for rational exponents | Can be complex |
Conclusion: Simplifying Radical Expressions Made Easy
Simplifying radical expressions like X 3 2 can be challenging, but with the right approach, it can be made easy. By understanding the different methods and their advantages and disadvantages, you can become proficient in simplifying radical expressions. Remember to practice regularly and apply these methods to various problems to solidify your understanding.
Now that you've read this article, we'd love to hear from you! Share your thoughts, ask questions, or provide feedback in the comments below. Don't forget to share this article with your friends and classmates who may benefit from it.
What is the difference between a radical expression and a rational expression?
+A radical expression is a mathematical expression that contains a square root or other roots, while a rational expression is a mathematical expression that contains only numbers and variables.
How do I know which method to use when simplifying radical expressions?
+The choice of method depends on the specific problem and the level of complexity. If the radicand is a perfect square, Method 1 is the most straightforward. If the radicand is not a perfect square, Method 2 or Method 3 may be more suitable.
Can I simplify radical expressions with variables?
+Yes, you can simplify radical expressions with variables using the same methods. However, you may need to apply additional techniques, such as factoring or cancelling, to simplify the expression further.