Simplify 72 In Radical Form In 3 Easy Steps

Quick Links :

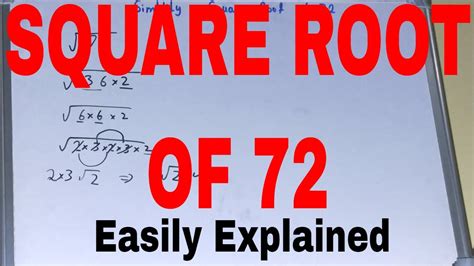
Simplifying radicals can be a challenging task, but with the right approach, it can be done in a few easy steps. In this article, we will simplify the radical √72 in 3 easy steps.

Step 1: Find the Prime Factorization of 72

To simplify the radical √72, we first need to find the prime factorization of 72. The prime factorization of 72 is 2^3 × 3^2. This means that 72 can be expressed as the product of its prime factors, which are 2 and 3.

Breaking Down 72 into Its Prime Factors

• 72 = 2 × 36
• 36 = 2 × 18
• 18 = 2 × 9
• 9 = 3 × 3

So, the prime factorization of 72 is 2^3 × 3^2.

Step 2: Identify the Perfect Square Factors

Now that we have the prime factorization of 72, we need to identify the perfect square factors. A perfect square factor is a factor that can be expressed as a square of an integer. In this case, we can see that 2^2 and 3^2 are both perfect square factors.

Identifying the Perfect Square Factors of 72

• 2^2 = 4
• 3^2 = 9

So, the perfect square factors of 72 are 4 and 9.

Step 3: Simplify the Radical

Now that we have identified the perfect square factors, we can simplify the radical √72. We can do this by factoring out the perfect square factors from the radical.

√72 = √(2^2 × 3^2 × 2) = √(4 × 9 × 2) = √(36 × 2) = 6√2

Therefore, the simplified form of √72 is 6√2.

Recap: Simplifying √72 in 3 Easy Steps

• Step 1: Find the prime factorization of 72 (2^3 × 3^2)
• Step 2: Identify the perfect square factors (2^2 and 3^2)
• Step 3: Simplify the radical by factoring out the perfect square factors (6√2)

By following these 3 easy steps, you can simplify any radical expression, including √72.

We hope this article has helped you understand how to simplify the radical √72 in 3 easy steps. If you have any questions or need further clarification, please don't hesitate to ask. Share this article with your friends and classmates to help them understand the concept of simplifying radicals.