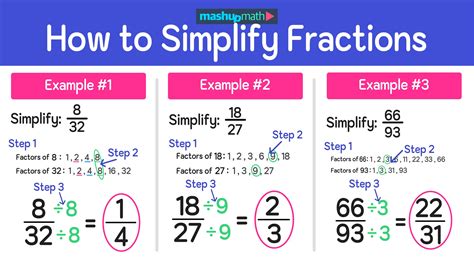
625 as a simplified fraction is a mathematical concept that involves expressing the decimal number 625 in its simplest fractional form. Understanding this concept is crucial in mathematics, particularly in algebra and geometry. In this article, we will delve into the world of fractions and explore how to simplify 625 as a fraction.
Importance of Simplifying Fractions
Fractions are an essential part of mathematics, and simplifying them is a critical skill to master. Simplifying fractions helps to reduce the complexity of mathematical expressions, making them easier to work with. It also enables us to identify equivalent fractions, which is vital in solving mathematical problems. In real-life scenarios, simplifying fractions is used in various applications, such as cooking, finance, and engineering.
Understanding the Concept of Equivalent Fractions
Before we dive into simplifying 625 as a fraction, it's essential to understand the concept of equivalent fractions. Equivalent fractions are fractions that have the same value but may have different numerators and denominators. For example, 1/2 and 2/4 are equivalent fractions because they both represent the same value. To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and denominator and divide both numbers by the GCD.
Simplifying 625 as a Fraction
To simplify 625 as a fraction, we need to find the prime factorization of 625. The prime factorization of 625 is 5 x 5 x 5 x 5 or 5^4. We can express 625 as a fraction by writing it as 625/1. However, this fraction is not in its simplest form. To simplify it, we need to find the GCD of 625 and 1, which is 1. Since the GCD is 1, we cannot simplify the fraction further.
Expressing 625 as a Fraction with a Denominator Other Than 1
Although we cannot simplify 625/1 further, we can express 625 as a fraction with a denominator other than 1. For example, we can write 625 as 625/1000. However, this fraction is not in its simplest form. To simplify it, we need to find the GCD of 625 and 1000, which is 125. Dividing both numbers by 125, we get 5/8.
Practical Applications of Simplifying 625 as a Fraction
Simplifying 625 as a fraction has several practical applications in real-life scenarios. For example, in cooking, we may need to simplify a recipe that involves 625 grams of ingredients. By expressing 625 as a fraction, we can easily scale down the recipe to smaller quantities. In finance, simplifying fractions is used in calculating interest rates and investment returns.
Real-Life Scenarios Where Simplifying 625 as a Fraction is Used
Simplifying 625 as a fraction is used in various real-life scenarios, such as:
- Cooking: Simplifying recipes that involve 625 grams of ingredients.
- Finance: Calculating interest rates and investment returns.
- Engineering: Measuring and calculating quantities in construction projects.
Conclusion: Mastering the Art of Simplifying Fractions
In conclusion, simplifying 625 as a fraction is a crucial mathematical concept that has various practical applications in real-life scenarios. By mastering the art of simplifying fractions, we can reduce the complexity of mathematical expressions, identify equivalent fractions, and solve mathematical problems with ease. Whether you're a student, teacher, or professional, understanding how to simplify fractions is an essential skill to acquire.
Take Action: Practice Simplifying Fractions
Now that you've learned the art of simplifying fractions, it's time to put your knowledge into practice. Try simplifying different fractions, including 625, to improve your mathematical skills. With practice and patience, you'll become a master of simplifying fractions in no time. Share your thoughts and experiences in the comments section below and help others improve their mathematical skills.
What is the importance of simplifying fractions?
+
Simplifying fractions is important because it helps to reduce the complexity of mathematical expressions, making them easier to work with. It also enables us to identify equivalent fractions, which is vital in solving mathematical problems.
How do you simplify a fraction?
+
To simplify a fraction, you need to find the greatest common divisor (GCD) of the numerator and denominator and divide both numbers by the GCD.
What are the practical applications of simplifying fractions?
+
Simplifying fractions has various practical applications in real-life scenarios, such as cooking, finance, and engineering.