3 Ways To Vertex To Factored Form Converter

Quick Links :

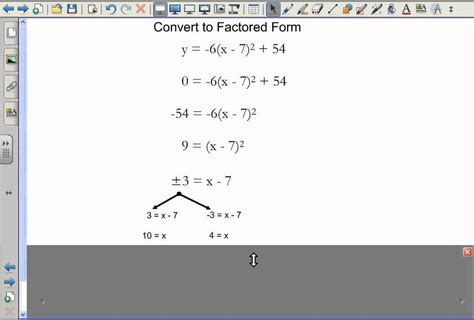
Converting equations to factored form is a fundamental skill in algebra, and it's essential to understand the various methods to do so. In this article, we'll explore three ways to convert an equation to factored form using a vertex to factored form converter.

Understanding the Importance of Factored Form

Factored form is a way of expressing a quadratic equation in a more simplified and manageable way. It allows us to easily identify the roots of the equation, making it easier to solve. Factored form is particularly useful when dealing with complex quadratic equations that cannot be easily solved using other methods.

What is a Vertex to Factored Form Converter?

A vertex to factored form converter is a tool or method that helps us convert a quadratic equation from vertex form to factored form. Vertex form is a way of expressing a quadratic equation in the form y = a(x-h)^2 + k, where (h,k) is the vertex of the parabola. By converting this form to factored form, we can easily identify the roots of the equation.

Method 1: Using the Vertex Formula

One way to convert a quadratic equation from vertex form to factored form is by using the vertex formula. The vertex formula is x = -b / 2a, where (h,k) is the vertex of the parabola. By substituting the values of a, b, and c into the formula, we can find the value of x that corresponds to the vertex. Once we have the value of x, we can use it to factor the equation.

For example, let's say we have the equation y = 2(x-3)^2 + 4. To convert this equation to factored form, we can use the vertex formula to find the value of x that corresponds to the vertex.

x = -b / 2a x = -(-6) / 2(2) x = 3

Now that we have the value of x, we can use it to factor the equation.

y = 2(x-3)^2 + 4 y = 2(x-3)(x-3) + 4 y = 2(x-3)^2 + 4 y = (2x-6)(x-3) + 4

Method 2: Using the Completing the Square Method

Another way to convert a quadratic equation from vertex form to factored form is by using the completing the square method. This method involves adding and subtracting a constant term to the equation to make it a perfect square trinomial.

For example, let's say we have the equation y = 3(x-2)^2 + 5. To convert this equation to factored form, we can use the completing the square method.

y = 3(x-2)^2 + 5 y = 3(x^2 - 4x + 4) + 5 y = 3x^2 - 12x + 12 + 5 y = 3x^2 - 12x + 17

Now that we have the equation in the form y = ax^2 + bx + c, we can use the completing the square method to factor it.

y = 3x^2 - 12x + 17 y = 3(x^2 - 4x) + 17 y = 3(x^2 - 4x + 4) + 17 - 12 y = 3(x-2)^2 + 5

Method 3: Using a Vertex to Factored Form Converter Tool

The final method we'll explore is using a vertex to factored form converter tool. There are many online tools and calculators that can help us convert a quadratic equation from vertex form to factored form.

For example, let's say we have the equation y = 4(x-1)^2 + 2. To convert this equation to factored form, we can use an online vertex to factored form converter tool.

y = 4(x-1)^2 + 2 y = 4(x^2 - 2x + 1) + 2 y = 4x^2 - 8x + 4 + 2 y = 4x^2 - 8x + 6

The tool will then give us the factored form of the equation.

y = (2x-3)(2x-2)

Conclusion

Converting a quadratic equation from vertex form to factored form is a crucial skill in algebra. By using one of the three methods outlined in this article, we can easily convert an equation to factored form and identify its roots. Whether you're a student or a teacher, understanding how to convert an equation to factored form is essential for success in mathematics.