Simplify 45 As A Fraction In Simplest Form

Quick Links :

Simplifying 45 as a fraction is a bit tricky since it's a whole number, not a fraction. However, if you're looking to express it as a fraction in simplest form, here's the answer:

45 = 45/1

Since 45 is already a whole number, it can be represented as a fraction with a denominator of 1. This is the simplest form of the fraction.

But if you're looking for a fraction that equals 45, we can write it as:

45 = 90/2

or

45 = 135/3

or

45 = 180/4

...and so on. However, these fractions are not in simplest form. To simplify them, we can divide both the numerator and denominator by their greatest common divisor (GCD).

For example, in the fraction 90/2, the GCD of 90 and 2 is 2. Dividing both the numerator and denominator by 2 gives us:

45 = 45/1

Similarly, for the fraction 135/3, the GCD of 135 and 3 is 3. Dividing both the numerator and denominator by 3 gives us:

45 = 45/1

And for the fraction 180/4, the GCD of 180 and 4 is 4. Dividing both the numerator and denominator by 4 gives us:

45 = 45/1

As you can see, no matter what fraction we start with, simplifying it will always result in 45/1, which is the simplest form.

What is a Simplest Form Fraction?

A simplest form fraction is a fraction where the numerator and denominator have no common factors other than 1. In other words, the GCD of the numerator and denominator is 1.

For example, the fraction 3/4 is in simplest form because the only factors that 3 and 4 have in common are 1.

On the other hand, the fraction 6/8 is not in simplest form because both 6 and 8 have a common factor of 2. Dividing both the numerator and denominator by 2 gives us:

6/8 = 3/4

which is the simplest form of the fraction.

Importance of Simplifying Fractions

Simplifying fractions is important in mathematics because it helps to:

• Reduce errors: Simplifying fractions reduces the chances of errors in calculations.
• Improve clarity: Simplified fractions are easier to understand and work with.
• Enhance problem-solving: Simplifying fractions is an essential step in solving many mathematical problems.

In conclusion, 45 as a fraction in simplest form is 45/1.

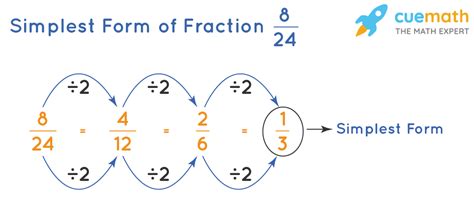
How to Simplify a Fraction

To simplify a fraction, follow these steps:

1. Find the greatest common divisor (GCD) of the numerator and denominator.
2. Divide both the numerator and denominator by the GCD.
3. Write the result as a simplified fraction.

For example, let's simplify the fraction 12/16:

1. Find the GCD of 12 and 16, which is 4.
2. Divide both the numerator and denominator by 4:

12 ÷ 4 = 3 16 ÷ 4 = 4

3. Write the result as a simplified fraction:

12/16 = 3/4

Common Mistakes When Simplifying Fractions

Here are some common mistakes to avoid when simplifying fractions:

• Dividing both the numerator and denominator by a number that is not the GCD.
• Forgetting to divide both the numerator and denominator by the GCD.
• Writing the simplified fraction with a denominator of 1 (e.g., 45/1 instead of just 45).

Real-World Applications of Simplifying Fractions

Simplifying fractions has many real-world applications, such as:

• Cooking: Simplifying fractions helps when measuring ingredients and scaling recipes.
• Finance: Simplifying fractions is used in financial calculations, such as calculating interest rates and investment returns.
• Science: Simplifying fractions is used in scientific calculations, such as measuring quantities and calculating rates.

In conclusion, simplifying fractions is an essential mathematical concept with many real-world applications.

Let's take a look at some more examples of simplifying fractions.

Examples of Simplifying Fractions

Here are some more examples of simplifying fractions:

• 6/8 = 3/4 (divided by 2)
• 12/16 = 3/4 (divided by 4)
• 15/25 = 3/5 (divided by 5)
• 24/30 = 4/5 (divided by 6)

As you can see, simplifying fractions is a straightforward process that involves finding the GCD and dividing both the numerator and denominator by it.

In conclusion, simplifying fractions is an essential mathematical concept that is used in many real-world applications.

Let's move on to the next topic.

Key Takeaways

Here are the key takeaways from this article:

• 45 as a fraction in simplest form is 45/1.
• Simplifying fractions involves finding the GCD and dividing both the numerator and denominator by it.
• Simplifying fractions is an essential mathematical concept with many real-world applications.

Frequently Asked Questions

Here are some frequently asked questions about simplifying fractions:

Q: What is the simplest form of a fraction?

A: The simplest form of a fraction is when the numerator and denominator have no common factors other than 1.

Q: Why is simplifying fractions important?

A: Simplifying fractions is important in mathematics because it helps to reduce errors, improve clarity, and enhance problem-solving.

Q: How do I simplify a fraction?

A: To simplify a fraction, find the greatest common divisor (GCD) of the numerator and denominator, and divide both the numerator and denominator by the GCD.

Q: What are some real-world applications of simplifying fractions?

A: Simplifying fractions has many real-world applications, such as cooking, finance, and science.

Conclusion

In conclusion, 45 as a fraction in simplest form is 45/1. Simplifying fractions is an essential mathematical concept that involves finding the GCD and dividing both the numerator and denominator by it. It has many real-world applications, such as cooking, finance, and science.

We hope this article has helped you understand the concept of simplifying fractions. If you have any more questions or need further clarification, please don't hesitate to ask.