4 Ways To Master Point-Slope Form Equations

Quick Links :

Unlocking the Power of Point-Slope Form Equations

Mastering point-slope form equations is a crucial milestone in algebra, enabling you to represent linear equations in a concise and intuitive way. This mathematical concept is a building block for more advanced algebraic techniques, and its applications are diverse, ranging from physics and engineering to economics and computer science. In this article, we will delve into the world of point-slope form equations, exploring their definition, benefits, and providing four practical ways to master them.

What are Point-Slope Form Equations?

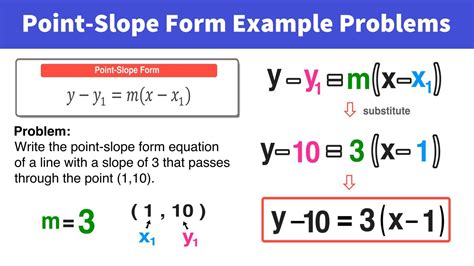
A point-slope form equation is a linear equation that represents a line on a coordinate plane. It is defined as y - y1 = m(x - x1), where (x1, y1) is a point on the line, and m is the slope of the line. The slope, m, represents the rate of change of the line, indicating how steep it is. The point-slope form is particularly useful when you know a point on the line and the slope, as it allows you to easily derive the equation of the line.

Benefits of Point-Slope Form Equations

Point-slope form equations offer several benefits that make them a valuable tool in algebra and various applications:

• Concise representation: Point-slope form equations provide a compact way to represent linear equations, making it easier to analyze and solve problems.
• Easy to derive: When you know a point on the line and the slope, it's straightforward to derive the equation of the line using the point-slope form.
• Versatile applications: Point-slope form equations have numerous applications in physics, engineering, economics, and computer science, making them a fundamental concept to master.

4 Ways to Master Point-Slope Form Equations

To master point-slope form equations, follow these four practical ways:

1. Understand the Concept

To master point-slope form equations, it's essential to understand the concept behind them. Start by grasping the definition of point-slope form equations and the role of the slope and point in the equation. Practice deriving the equation of a line using the point-slope form, given a point and the slope.

2. Practice with Simple Examples

Practice makes perfect! Start with simple examples, such as finding the equation of a line given a point and the slope. Use online resources or worksheets to practice solving point-slope form equations. As you become more confident, move on to more complex examples.

3. Visualize the Equations

Visualizing point-slope form equations can help you better understand the concept. Use graphing software or a graphing calculator to visualize the lines represented by point-slope form equations. This will help you connect the equation to the graphical representation of the line.

4. Apply to Real-World Problems

Apply point-slope form equations to real-world problems to reinforce your understanding. For example, you can use point-slope form equations to model the relationship between two variables in physics, economics, or engineering. This will help you see the practical value of point-slope form equations and make the concept more memorable.

Additional Tips

• Use online resources: There are many online resources, such as video tutorials and interactive quizzes, that can help you master point-slope form equations.
• Join a study group: Joining a study group or finding a study partner can help you stay motivated and get help when you need it.
• Take practice quizzes: Regular practice quizzes can help you assess your understanding and identify areas where you need to focus your efforts.

Conclusion

Mastering point-slope form equations is an essential milestone in algebra, enabling you to represent linear equations in a concise and intuitive way. By following the four practical ways outlined in this article, you can develop a deep understanding of point-slope form equations and apply them to real-world problems. Remember to practice regularly, use online resources, and join a study group to reinforce your learning.