2 Easy Ways To Convert 2x 5y 10 To Slope Intercept

Quick Links :

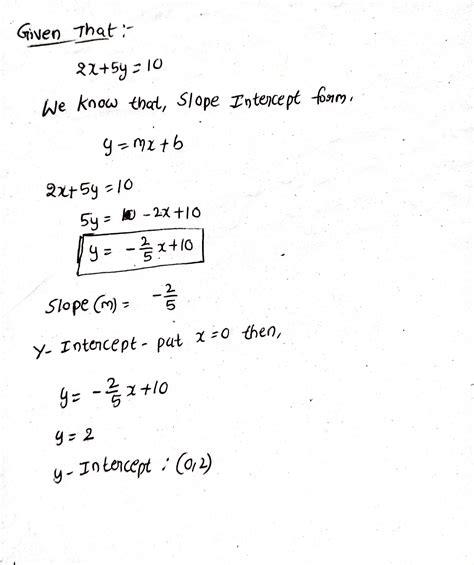
Converting a linear equation from standard form to slope-intercept form can seem daunting, but it's actually quite straightforward. In this article, we'll explore two easy ways to convert the equation 2x + 5y = 10 to slope-intercept form.

Why Convert to Slope-Intercept Form?

Before we dive into the conversion methods, let's quickly discuss why we want to convert to slope-intercept form in the first place. The slope-intercept form, y = mx + b, is often preferred because it clearly shows the slope (m) and the y-intercept (b) of the line. This makes it easier to graph the line, find the x-intercept, and identify key features of the line.

Method 1: Rearranging the Equation

One way to convert 2x + 5y = 10 to slope-intercept form is to rearrange the equation to isolate y.

Start by subtracting 2x from both sides of the equation:

2x + 5y = 10 5y = -2x + 10

Next, divide both sides by 5 to solve for y:

y = (-2/5)x + 2

And that's it! We've successfully converted the equation to slope-intercept form.

Method 2: Using the Slope-Intercept Formula

Another way to convert 2x + 5y = 10 to slope-intercept form is to use the slope-intercept formula, y = mx + b. To do this, we need to identify the slope (m) and the y-intercept (b).

The slope (m) can be found by looking at the coefficient of x. In this case, the coefficient of x is -2/5, so the slope is -2/5.

The y-intercept (b) can be found by looking at the constant term. In this case, the constant term is 2, so the y-intercept is 2.

Now that we have the slope and y-intercept, we can plug them into the slope-intercept formula:

y = (-2/5)x + 2

As you can see, both methods yield the same result.

Key Takeaways

Converting a linear equation from standard form to slope-intercept form is a crucial skill in algebra and graphing. By rearranging the equation or using the slope-intercept formula, you can easily convert equations like 2x + 5y = 10 to slope-intercept form. Remember to identify the slope and y-intercept, and use them to write the equation in slope-intercept form.

Tips and Variations

• When rearranging the equation, make sure to perform the operations in the correct order (e.g., subtract 2x from both sides, then divide both sides by 5).
• When using the slope-intercept formula, make sure to identify the slope and y-intercept correctly.
• Practice converting different types of linear equations to slope-intercept form, such as equations with fractions or decimals.

Real-World Applications

Converting linear equations to slope-intercept form has numerous real-world applications, including:

• Graphing: Slope-intercept form makes it easy to graph lines, which is essential in fields like engineering, physics, and economics.
• Linear Programming: Slope-intercept form is used to model linear programming problems, which involve optimizing a linear objective function subject to constraints.
• Data Analysis: Slope-intercept form can be used to analyze and model real-world data, such as population growth or financial trends.

Example Problems

Try converting the following equations to slope-intercept form:

• 3x + 2y = 12
• x - 4y = -8
• 2x - 3y = 15

Conclusion

Converting linear equations to slope-intercept form is a valuable skill that can be applied to a wide range of mathematical and real-world problems. By using the methods outlined in this article, you'll be well on your way to becoming a master of linear equations. So, next time you encounter an equation like 2x + 5y = 10, you'll know exactly how to convert it to slope-intercept form.