Fraction Conversion Simplified: A Comprehensive Guide

Fractions are a fundamental concept in mathematics, but they can be intimidating, especially when it comes to conversion. Whether you're a student, teacher, or simply someone who wants to improve their math skills, understanding fraction conversion is essential. In this article, we'll break down the basics of fraction conversion, provide practical examples, and offer tips to make the process easier.
Why is Fraction Conversion Important?
Fraction conversion is a crucial skill in mathematics, as it allows us to express fractions in different forms, making it easier to solve problems and compare values. For instance, converting a fraction to a decimal or percentage can help you understand the proportion of a whole. Moreover, fraction conversion is used in various real-life applications, such as cooking, finance, and science.
Understanding the Basics of Fraction Conversion

Before diving into the conversion process, it's essential to understand the basics of fractions. A fraction consists of two parts: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into.
Types of Fraction Conversion
There are several types of fraction conversion, including:
- Simplifying fractions: reducing a fraction to its simplest form by dividing both the numerator and denominator by the greatest common divisor (GCD).
- Converting fractions to decimals: expressing a fraction as a decimal by dividing the numerator by the denominator.
- Converting fractions to percentages: expressing a fraction as a percentage by dividing the numerator by the denominator and multiplying by 100.
- Converting mixed numbers to improper fractions: converting a mixed number (a combination of a whole number and a fraction) to an improper fraction (a fraction with a numerator greater than the denominator).
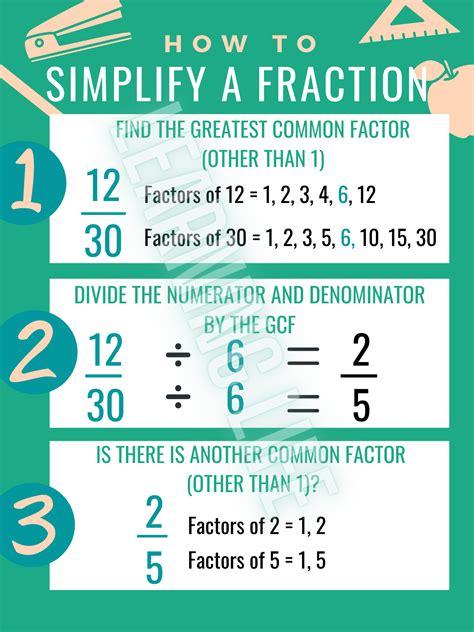
Simplifying Fractions

Simplifying fractions is an essential skill in fraction conversion. To simplify a fraction, you need to find the greatest common divisor (GCD) of the numerator and denominator and divide both numbers by the GCD.
For example, let's simplify the fraction 6/8:
- Find the GCD of 6 and 8, which is 2.
- Divide both numbers by the GCD: 6 ÷ 2 = 3 and 8 ÷ 2 = 4.
- The simplified fraction is 3/4.
Converting Fractions to Decimals
Converting fractions to decimals is a straightforward process. Simply divide the numerator by the denominator, and you'll get the decimal equivalent.
For example, let's convert the fraction 3/4 to a decimal:
- Divide the numerator by the denominator: 3 ÷ 4 = 0.75.
- The decimal equivalent of 3/4 is 0.75.
Converting Fractions to Percentages

Converting fractions to percentages is a bit more complex, but still a manageable process. To convert a fraction to a percentage, divide the numerator by the denominator and multiply by 100.
For example, let's convert the fraction 3/4 to a percentage:
- Divide the numerator by the denominator: 3 ÷ 4 = 0.75.
- Multiply by 100: 0.75 × 100 = 75%.
- The percentage equivalent of 3/4 is 75%.
Converting Mixed Numbers to Improper Fractions
Converting mixed numbers to improper fractions is a useful skill in fraction conversion. To convert a mixed number to an improper fraction, multiply the whole number by the denominator and add the numerator.
For example, let's convert the mixed number 2 3/4 to an improper fraction:
- Multiply the whole number by the denominator: 2 × 4 = 8.
- Add the numerator: 8 + 3 = 11.
- The improper fraction equivalent of 2 3/4 is 11/4.
Practical Applications of Fraction Conversion

Fraction conversion has numerous practical applications in real-life scenarios. Here are a few examples:
- Cooking: When following a recipe, you may need to convert fractions to decimals or percentages to adjust the ingredient quantities.
- Finance: When calculating interest rates or investment returns, you may need to convert fractions to decimals or percentages.
- Science: When measuring quantities or calculating ratios, you may need to convert fractions to decimals or percentages.
Tips and Tricks for Easy Fraction Conversion
Here are some tips and tricks to make fraction conversion easier:
- Use online tools: There are many online tools and calculators that can help you convert fractions quickly and accurately.
- Practice, practice, practice: The more you practice fraction conversion, the more comfortable you'll become with the process.
- Use real-life examples: Try to relate fraction conversion to real-life scenarios to make the process more meaningful and engaging.
What is the purpose of fraction conversion?
+Fraction conversion is used to express fractions in different forms, making it easier to solve problems and compare values.
How do I simplify a fraction?
+To simplify a fraction, find the greatest common divisor (GCD) of the numerator and denominator and divide both numbers by the GCD.
How do I convert a fraction to a decimal?
+To convert a fraction to a decimal, divide the numerator by the denominator.
In conclusion, fraction conversion is a fundamental skill in mathematics that can seem intimidating at first, but with practice and the right tools, it can become second nature. By understanding the basics of fraction conversion, simplifying fractions, and converting fractions to decimals and percentages, you'll be able to tackle a wide range of mathematical problems with confidence.