0.56 As A Fraction In Simplest Form

Quick Links :

Converting decimals to fractions is an essential skill in mathematics, and it can be a bit tricky at times. However, with the right approach, you can easily convert 0.56 to a fraction in its simplest form.

To start with, let's understand what a decimal is. A decimal is a way of expressing a number using a point (.) to separate the whole part from the fractional part. In the case of 0.56, the whole part is 0, and the fractional part is 56.

Now, to convert 0.56 to a fraction, we need to find the equivalent fraction with a denominator that is a power of 10. Since 0.56 has two decimal places, we can multiply it by 100 to get rid of the decimal point.

0.56 x 100 = 56

Now, we can write 56 as a fraction with a denominator of 100:

56/100

However, this fraction is not in its simplest form. To simplify it, we need to find the greatest common divisor (GCD) of 56 and 100. The GCD is the largest number that divides both 56 and 100 without leaving a remainder.

The GCD of 56 and 100 is 4. So, we can divide both the numerator and the denominator by 4 to simplify the fraction:

56 ÷ 4 = 14 100 ÷ 4 = 25

Now, we have the simplified fraction:

14/25

So, 0.56 as a fraction in its simplest form is 14/25.

Why is it important to simplify fractions?

Simplifying fractions is essential in mathematics because it helps us to:

• Reduce errors: Simplifying fractions reduces the risk of errors when performing calculations.
• Improve readability: Simplified fractions are easier to read and understand.
• Enhance problem-solving: Simplifying fractions makes it easier to solve problems and equations.
• Develop critical thinking: Simplifying fractions requires critical thinking and problem-solving skills.

How to simplify fractions?

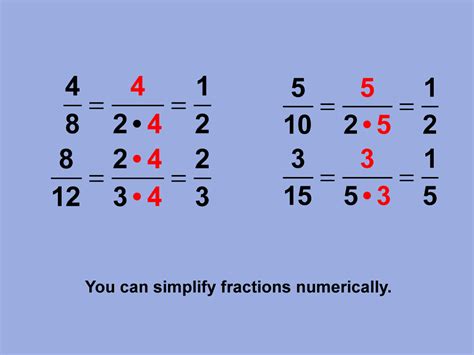
Simplifying fractions involves finding the greatest common divisor (GCD) of the numerator and the denominator and dividing both numbers by the GCD.

Here are the steps to simplify a fraction:

1. Find the GCD of the numerator and the denominator.
2. Divide the numerator and the denominator by the GCD.
3. Write the simplified fraction.

For example, let's simplify the fraction 12/18:

1. Find the GCD of 12 and 18: GCD(12, 18) = 6
2. Divide the numerator and the denominator by the GCD: 12 ÷ 6 = 2, 18 ÷ 6 = 3
3. Write the simplified fraction: 2/3

Types of fractions

There are several types of fractions, including:

• Proper fractions: A fraction where the numerator is less than the denominator.
• Improper fractions: A fraction where the numerator is greater than or equal to the denominator.
• Mixed fractions: A fraction that consists of a whole number and a proper fraction.
• Equivalent fractions: Fractions that have the same value but different numerators and denominators.

Understanding the different types of fractions is essential in mathematics, and simplifying fractions is a critical skill that can help you to solve problems and equations more efficiently.

Real-world applications of fractions

Fractions have numerous real-world applications, including:

• Cooking and recipe measurements
• Music and rhythm
• Finance and accounting
• Science and engineering
• Architecture and design

In cooking, fractions are used to measure ingredients and scale recipes. In music, fractions are used to represent time signatures and rhythms. In finance, fractions are used to calculate interest rates and investments.

Understanding fractions and how to simplify them is essential in a wide range of real-world applications.

Conclusion

In conclusion, converting 0.56 to a fraction in its simplest form is a straightforward process that involves finding the equivalent fraction with a denominator that is a power of 10 and simplifying it by dividing both the numerator and the denominator by the greatest common divisor.

Simplifying fractions is an essential skill in mathematics that can help you to solve problems and equations more efficiently, and it has numerous real-world applications in cooking, music, finance, science, and architecture.

We hope this article has helped you to understand the concept of fractions and how to simplify them. If you have any questions or need further clarification, please don't hesitate to ask.