5 Ways To Convert Y Intercept To Standard Form

Quick Links :

Hide

The y-intercept form of a linear equation is a fundamental concept in mathematics, particularly in algebra and geometry. It provides a convenient way to express a line in terms of its slope and y-intercept. However, there are situations where it's necessary to convert the y-intercept form to the standard form of a linear equation. In this article, we'll explore five ways to achieve this conversion.

Understanding the Y-Intercept Form

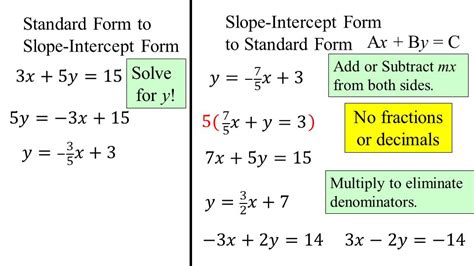
The y-intercept form of a linear equation is given by:

y = mx + b

where m is the slope of the line and b is the y-intercept. This form is useful for graphing lines, as it provides the y-coordinate of the point where the line intersects the y-axis.

Why Convert to Standard Form?

The standard form of a linear equation, also known as the general form, is given by:

Ax + By = C

where A, B, and C are constants. Converting the y-intercept form to standard form is essential in various mathematical and real-world applications, such as:

• Finding the equation of a line given two points
• Determining the intersection of two lines
• Solving systems of linear equations

Method 1: Rearranging the Equation

One way to convert the y-intercept form to standard form is by rearranging the equation. To do this, simply move the x-term to the left side of the equation and change the sign of the constant term:

y = mx + b mx + y = b

Now, multiply both sides by -1 to get:

-mx - y = -b

This is the standard form of the equation.

Method 2: Using the Slope-Intercept Form

Another way to convert the y-intercept form to standard form is by using the slope-intercept form. The slope-intercept form is given by:

y - y1 = m(x - x1)

where (x1, y1) is a point on the line. To convert to standard form, substitute the values of x1 and y1 into the equation and simplify:

y - b = m(x - 0) y - b = mx mx - y = -b

This is the standard form of the equation.

Method 3: Using the Point-Slope Form

The point-slope form of a linear equation is given by:

y - y1 = m(x - x1)

where (x1, y1) is a point on the line. To convert to standard form, substitute the values of x1 and y1 into the equation and simplify:

y - b = m(x - 0) y - b = mx mx - y = -b

This is the standard form of the equation.

Method 4: Using Algebraic Manipulation

Another way to convert the y-intercept form to standard form is by using algebraic manipulation. Multiply both sides of the equation by -1 and add y to both sides:

y = mx + b -mx - y = -b

Now, add x to both sides and simplify:

-mx - y + x = -b + x x - mx - y = x - b

This is the standard form of the equation.

Method 5: Using a Graphing Calculator

A graphing calculator can also be used to convert the y-intercept form to standard form. Simply enter the equation in the calculator and use the "Equation" or "Form" feature to convert it to standard form.

Conclusion

Converting the y-intercept form to standard form is an essential skill in mathematics. In this article, we've explored five ways to achieve this conversion: rearranging the equation, using the slope-intercept form, using the point-slope form, using algebraic manipulation, and using a graphing calculator. By mastering these methods, you'll be able to tackle a wide range of mathematical problems with confidence.

We hope this article has been informative and helpful. If you have any questions or comments, please feel free to share them below.