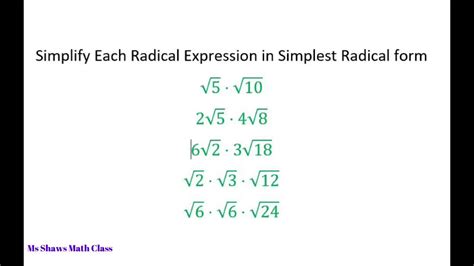
Writing Expressions In Radical Form Made Easy

Quick Links :

Writing expressions in radical form can seem like a daunting task, especially for those who are new to mathematics. However, with the right approach and understanding, it can be made easy and even enjoyable. In this article, we will explore the world of radicals and provide you with a comprehensive guide on how to write expressions in radical form.

What are Radicals?

Radicals, also known as roots, are mathematical symbols used to represent the nth root of a number. The most common type of radical is the square root, which is represented by the symbol √. Radicals are used to simplify complex mathematical expressions and to solve equations that involve roots.

Why are Radicals Important?

Radicals are an essential part of mathematics, and they have numerous applications in various fields, including physics, engineering, and computer science. They are used to solve problems that involve rates, ratios, and proportions, and they are a crucial part of algebra and calculus.

How to Write Expressions in Radical Form

Writing expressions in radical form involves converting mathematical expressions into a form that uses radicals instead of rational exponents. Here are the steps to follow:

• Identify the base and the exponent of the expression.
• Determine the index of the radical, which is the same as the denominator of the rational exponent.
• Write the base inside the radical symbol and the index outside the symbol.
• Simplify the expression by evaluating the radical.

For example, to write the expression 16^(1/2) in radical form, we would follow these steps:

• Identify the base (16) and the exponent (1/2).
• Determine the index of the radical, which is 2.
• Write the base inside the radical symbol and the index outside the symbol: √16.
• Simplify the expression by evaluating the radical: √16 = 4.

Rules for Writing Expressions in Radical Form

There are several rules to follow when writing expressions in radical form:

• The base must be a non-negative real number.
• The index must be a positive integer.
• The expression must be simplified by evaluating the radical.
• The radical symbol must be used only when the index is 2 or higher.

For example, the expression 4^(1/3) can be written in radical form as ∛4, but the expression 4^(1/1) cannot be written in radical form because the index is 1.

Common Mistakes to Avoid

When writing expressions in radical form, there are several common mistakes to avoid:

• Using the radical symbol with a negative base.
• Using the radical symbol with a fractional exponent that has a denominator other than a positive integer.
• Failing to simplify the expression by evaluating the radical.
• Using the radical symbol with an index of 1.

For example, the expression -4^(1/2) cannot be written in radical form because the base is negative. Similarly, the expression 4^(1/3) cannot be written in radical form as √[3]4 because the index is not 2 or higher.

Practical Applications of Radicals

Radicals have numerous practical applications in various fields, including physics, engineering, and computer science. Here are a few examples:

• Physics: Radicals are used to solve problems involving rates, ratios, and proportions, such as calculating the distance traveled by an object under constant acceleration.
• Engineering: Radicals are used to design and optimize systems, such as calculating the stress on a beam or the load on a bridge.
• Computer Science: Radicals are used in algorithms for solving complex mathematical problems, such as finding the roots of a polynomial equation.

Real-World Examples of Radicals

Here are a few real-world examples of radicals:

• The square root of 2 is an irrational number that is used in construction to calculate the length of the diagonal of a square.
• The cube root of 64 is used in physics to calculate the volume of a cube.
• The fourth root of 256 is used in computer science to calculate the roots of a polynomial equation.

Conclusion: Take Control of Your Math Skills

Writing expressions in radical form can seem daunting at first, but with practice and patience, it can become second nature. By following the rules and guidelines outlined in this article, you can take control of your math skills and solve complex problems with ease. Remember to simplify expressions by evaluating the radical, avoid common mistakes, and apply radicals to real-world problems. With time and practice, you will become proficient in writing expressions in radical form and solving complex mathematical problems.

We hope this article has been helpful in making writing expressions in radical form easy and enjoyable. If you have any questions or comments, please share them with us below.