Understanding the Standard Form Equation of a Circle

The standard form equation of a circle is a mathematical concept that is essential for understanding various geometric and algebraic principles. In this article, we will delve into the world of circles, exploring the standard form equation, its significance, and how to work with it. Whether you are a student, teacher, or simply someone interested in mathematics, this article aims to provide a comprehensive and easy-to-understand guide to the standard form equation of a circle.
What is the Standard Form Equation of a Circle?
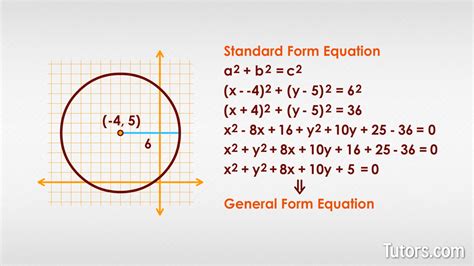
The standard form equation of a circle is given by:
(x - h)^2 + (y - k)^2 = r^2
where (h, k) represents the coordinates of the center of the circle, and r is the radius of the circle.
Breaking Down the Standard Form Equation

To fully understand the standard form equation, let's break it down into its components:
- (x - h)^2: This term represents the horizontal distance between the center of the circle and any point on the circle.
- (y - k)^2: This term represents the vertical distance between the center of the circle and any point on the circle.
- r^2: This term represents the square of the radius of the circle.
Significance of the Standard Form Equation
The standard form equation of a circle is significant in various mathematical and real-world applications. Some of the key reasons include:
- Graphing Circles: The standard form equation is used to graph circles on a coordinate plane.
- Finding the Center and Radius: The equation can be used to find the center and radius of a circle, given certain information about the circle.
- Circle-Circle Intersection: The standard form equation is used to find the intersection points of two or more circles.
Working with the Standard Form Equation

Here are some examples of working with the standard form equation:
- Example 1: Find the center and radius of the circle given by the equation (x - 2)^2 + (y - 3)^2 = 16.
- Example 2: Graph the circle given by the equation (x + 1)^2 + (y - 2)^2 = 25.
Steps to Graph a Circle using the Standard Form Equation
To graph a circle using the standard form equation, follow these steps:
- Identify the center of the circle (h, k).
- Identify the radius of the circle (r).
- Plot the center of the circle on the coordinate plane.
- Draw a circle with the radius (r) centered at the point (h, k).
Real-World Applications of the Standard Form Equation

The standard form equation of a circle has various real-world applications, including:
- GPS Navigation: The equation is used in GPS navigation systems to calculate distances and directions between locations.
- Medical Imaging: The equation is used in medical imaging techniques such as MRI and CT scans to reconstruct images of the body.
- Architecture: The equation is used in architecture to design and build circular structures such as domes and arches.
Common Mistakes to Avoid
When working with the standard form equation, it's essential to avoid common mistakes such as:
- Forgetting to square the radius: Remember to square the radius (r) when plugging it into the equation.
- Misidentifying the center: Make sure to correctly identify the center of the circle (h, k) when graphing the circle.
Conclusion
In conclusion, the standard form equation of a circle is a fundamental concept in mathematics that has various real-world applications. By understanding the equation and its components, you can graph circles, find the center and radius, and solve problems involving circle-circle intersection. Remember to avoid common mistakes and practice working with the equation to become proficient in using it.
What is the standard form equation of a circle?
+The standard form equation of a circle is given by (x - h)^2 + (y - k)^2 = r^2, where (h, k) represents the coordinates of the center of the circle, and r is the radius of the circle.
How do I graph a circle using the standard form equation?
+To graph a circle using the standard form equation, identify the center of the circle (h, k), identify the radius of the circle (r), plot the center of the circle on the coordinate plane, and draw a circle with the radius (r) centered at the point (h, k).
What are some real-world applications of the standard form equation of a circle?
+The standard form equation of a circle has various real-world applications, including GPS navigation, medical imaging, and architecture.