15 As A Fraction In Simplest Form

Quick Links :

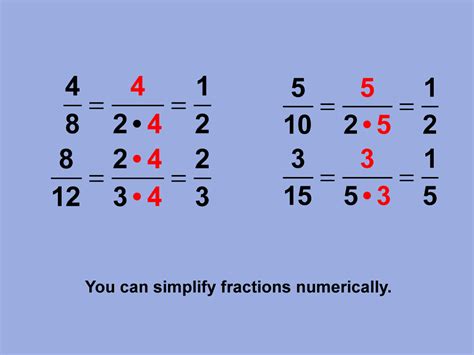
The concept of fractions is a fundamental aspect of mathematics, allowing us to express part of a whole as a numerical value. When we consider the number 15 as a fraction in its simplest form, we are essentially looking at how it can be represented as a ratio of two integers.

Understanding Fractions

A fraction is typically written in the format a/b, where 'a' represents the numerator (the top number) and 'b' represents the denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into.

Converting 15 to a Fraction

To convert the number 15 into a fraction, we need to consider its simplest form. The simplest form of a fraction is when the numerator and denominator have no common factors other than 1.

The number 15 can be written as a fraction with a denominator of 1, which would be 15/1. However, this is not the only way to represent 15 as a fraction. We can also express it as 15/1 = 30/2 = 45/3 = 60/4, and so on.

Simplifying the Fraction

However, when we simplify a fraction, we aim to find the ratio with the smallest possible numerator and denominator while maintaining the same value. In the case of 15, we can simplify it by dividing both the numerator and denominator by their greatest common divisor (GCD), which is 1.

Therefore, the simplest form of 15 as a fraction is 15/1.

Benefits of Simplifying Fractions

Simplifying fractions offers several benefits, including:

• Easier calculations: Simplified fractions make it easier to perform arithmetic operations such as addition, subtraction, multiplication, and division.
• Improved clarity: Simplifying fractions helps to eliminate unnecessary complexity, making it easier to understand and work with the numbers.
• Enhanced accuracy: By reducing fractions to their simplest form, we minimize the risk of errors and ensure more accurate calculations.

Real-World Applications of Fractions

Fractions are used in various real-world scenarios, such as:

• Measuring ingredients for cooking and baking
• Calculating proportions for art and design
• Determining probabilities in statistics and finance
• Expressing ratios in science and engineering

Common Challenges with Fractions

Some common challenges people face when working with fractions include:

• Difficulty in understanding equivalent ratios
• Struggling to simplify complex fractions
• Challenges in adding and subtracting fractions with unlike denominators
• Inability to convert between mixed numbers and improper fractions

Overcoming Challenges with Fractions

To overcome these challenges, it's essential to practice working with fractions regularly. Here are some tips to help you improve your skills:

• Start with simple fractions and gradually move on to more complex ones
• Use visual aids such as diagrams and charts to help you understand equivalent ratios
• Practice simplifying fractions by finding the greatest common divisor (GCD)
• Break down complex fractions into simpler ones to make calculations easier

Conclusion: Mastering Fractions

Mastering fractions requires practice, patience, and persistence. By understanding the concept of fractions, simplifying them, and applying them to real-world scenarios, you can overcome common challenges and become proficient in working with fractions.

If you have any questions or would like to share your experiences with fractions, please feel free to comment below.