5 Ways To Write Line Equations In Standard Form

Quick Links :

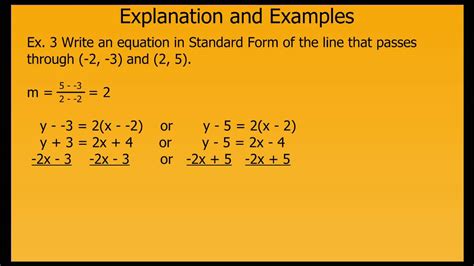
Writing line equations in standard form is a fundamental concept in mathematics, particularly in algebra and geometry. The standard form of a linear equation is Ax + By = C, where A, B, and C are integers, and A is non-negative. In this article, we will explore five ways to write line equations in standard form.

Understanding the Importance of Standard Form

Writing line equations in standard form is essential in mathematics because it provides a consistent and clear way to express linear relationships. It is particularly useful when working with systems of equations, graphing lines, and solving linear inequalities. Moreover, standard form is a crucial concept in various real-world applications, such as physics, engineering, and economics.

Method 1: Converting Slope-Intercept Form to Standard Form

The slope-intercept form of a linear equation is y = mx + b, where m is the slope and b is the y-intercept. To convert this form to standard form, we need to isolate x and y on opposite sides of the equation.

For example, consider the slope-intercept form equation y = 2x - 3. To convert it to standard form, we can rewrite it as:

2x - y = 3

This equation is now in standard form, with A = 2, B = -1, and C = 3.

Step-by-Step Instructions

1. Write the slope-intercept form equation.
2. Add or subtract the same value to both sides of the equation to isolate x and y.
3. Rearrange the equation to put it in the standard form Ax + By = C.

Method 2: Converting Point-Slope Form to Standard Form

The point-slope form of a linear equation is y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope. To convert this form to standard form, we need to simplify the equation and isolate x and y.

For example, consider the point-slope form equation y - 2 = 3(x - 1). To convert it to standard form, we can rewrite it as:

3x - y = 1

This equation is now in standard form, with A = 3, B = -1, and C = 1.

Step-by-Step Instructions

1. Write the point-slope form equation.
2. Simplify the equation by combining like terms.
3. Rearrange the equation to put it in the standard form Ax + By = C.

Method 3: Writing Equations in Standard Form from Graphs

We can also write line equations in standard form by using graphs. To do this, we need to identify the x-intercept and y-intercept of the line.

For example, consider the graph of a line with x-intercept (2, 0) and y-intercept (0, 3). The equation of this line in standard form is:

2x + 3y = 6

Step-by-Step Instructions

1. Identify the x-intercept and y-intercept of the line.
2. Write the equation in the form x/a + y/b = 1, where a is the x-intercept and b is the y-intercept.
3. Multiply both sides of the equation by the least common multiple of a and b to put it in the standard form Ax + By = C.

Method 4: Converting Parametric Equations to Standard Form

Parametric equations are a set of equations that express the x and y coordinates of a point in terms of a third variable, usually t. To convert parametric equations to standard form, we need to eliminate the parameter t.

For example, consider the parametric equations x = 2t + 1 and y = 3t - 2. To convert these equations to standard form, we can rewrite them as:

2x - 3y = 7

This equation is now in standard form, with A = 2, B = -3, and C = 7.

Step-by-Step Instructions

1. Write the parametric equations.
2. Solve for t in one of the equations.
3. Substitute the expression for t into the other equation.
4. Rearrange the equation to put it in the standard form Ax + By = C.

Method 5: Writing Equations in Standard Form from Word Problems

We can also write line equations in standard form by using word problems. To do this, we need to identify the key information in the problem and translate it into an equation.

For example, consider the following word problem: "Tom has $120 to spend on concert tickets and souvenirs. Tickets cost $20 each, and souvenirs cost $10 each. How many tickets and souvenirs can Tom buy?"

Let x be the number of tickets and y be the number of souvenirs. The equation of this problem in standard form is:

20x + 10y = 120

Step-by-Step Instructions

1. Read the word problem carefully and identify the key information.
2. Let x and y be the variables in the problem.
3. Write an equation that represents the problem.
4. Rearrange the equation to put it in the standard form Ax + By = C.

We hope this article has helped you understand the different ways to write line equations in standard form. Remember to practice each method to become more proficient in writing line equations in standard form.

Now it's your turn! Share your favorite method for writing line equations in standard form in the comments below. Don't forget to like and share this article with your friends and classmates.